Answered step by step
Verified Expert Solution
Question
1 Approved Answer
If f : P Q is onto (f[P] = Q), and X is a partition of Q. Let Y = {f-1 [x] | X
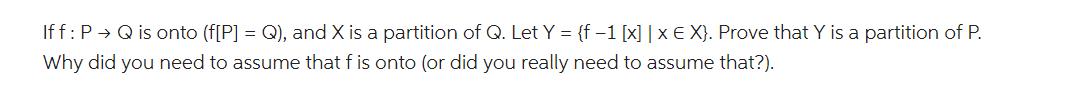
If f : P Q is onto (f[P] = Q), and X is a partition of Q. Let Y = {f-1 [x] | X E X). Prove that Y is a partition of P. Why did you need to assume that f is onto (or did you really need to assume that?).
Step by Step Solution
★★★★★
3.41 Rating (145 Votes )
There are 3 Steps involved in it
Step: 1
To prove that Y is a partition of P we need to show two things 1 Y is a collection of nonempty subsets of P and 2 the subsets in are pairwise disjoint ...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Introduction to Probability
Authors: Mark Daniel Ward, Ellen Gundlach
1st edition
716771098, 978-1319060893, 1319060897, 978-0716771098
