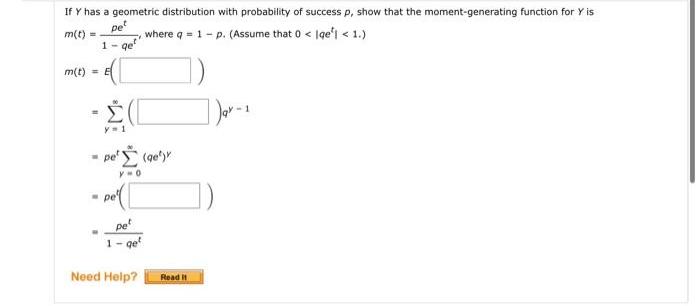
If Y has a geometric distribution with probability of success p, show that the moment-generating function for Y is pet m(t)= where q = 1- p. (Assume that 0 < |qe| < 1.) 1- m(t) = E get - ( y -pet y o 1- (ge) get Need Help? Read It ])ar-1 6. [-/5 Points] MY NOTES ASK YOUR TEACHER PRACTICE ANOTHER Find the distributions of the random variables that have each of the following moment generating functions. (a) m(e) = [(-)e + ()] DETAILS WACKERLYSTAT7 3.9.153. The distribution is -Select-, with n= e 2-e The distribution is -Select-, with p= (b) m(t)= (c) m(t) = e(e-1) The distribution is --Select-, with = Need Help? Read It and p =