Im going to attach the homework I need help in, I really need help with the explanations of this, thank you tutors in advance!

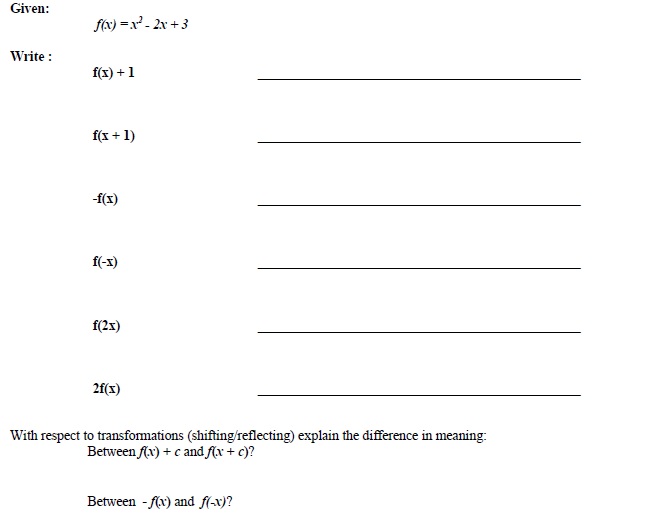
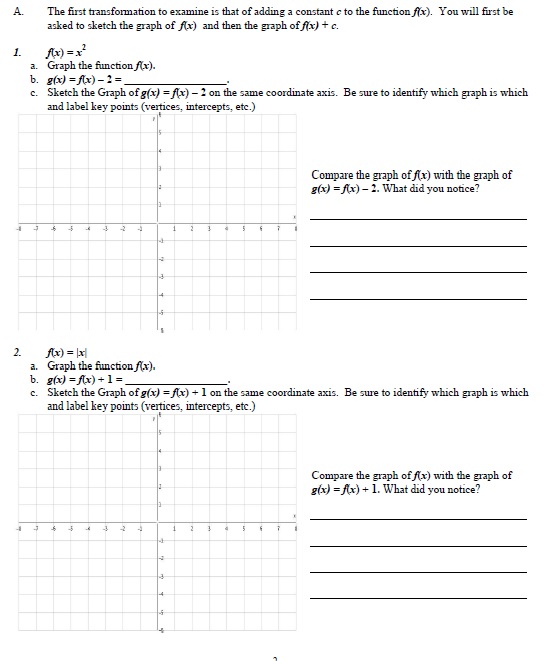
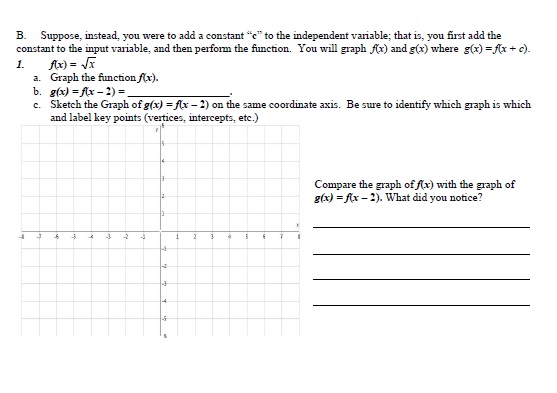
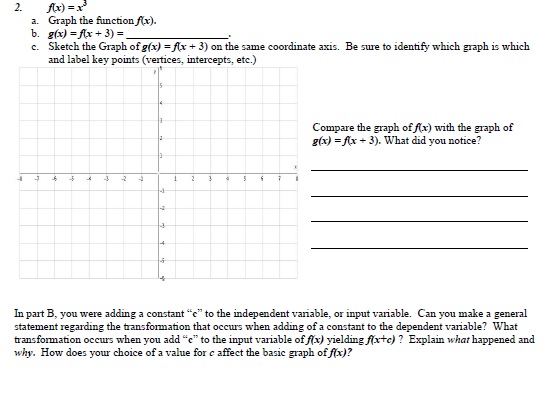
Given: f() =x - 2x+3 Write : f(x) + 1 f(x + 1) -f(x) f(-x) f(2x) 2f(x) With respect to transformations (shifting reflecting) explain the difference in meaning: Between f(x) + c and f(x + c)? Between - f(x) and f(-x)?A. The first transformation to examine is that of adding a constant c to the function fix). You will first be asked to sketch the graph of f(x) and then the graph of fix) + c. 1. Ax) = x a. Graph the function f(x). b. g(x) =/(x) - 1= c. Sketch the Graph of g(x) =/(x) - 1 on the same coordinate axis. Be sure to identify which graph is which and label key points (vertices, intercepts, etc.) Compare the graph of (x) with the graph of g(x) =/(x) - 1. What did you notice? 2 2. f(x) = x 2. Graph the function f(x). b. g(x) =/(x) +1= c. Sketch the Graph of g(x) =f(x) + 1 on the same coordinate axis. Be sure to identify which graph is which and label key points (vertices, intercepts, etc.) Compare the graph of A(x) with the graph of g(x) = A(x) + 1. What did you notice? -2 2B. Suppose, instead, you were to add a constant "c" to the independent variable; that is, you first add the constant to the iput variable, and then perform the function. You will graph /(x) and g(x) where g(x) =/(x + c). 1. Ax) = Vx a. Graph the function /(x). b. g(x) =/(x -1) = c. Sketch the Graph of g(x) =(x -1) on the same coordinate axis. Be sure to identify which graph is which and label key points (vertices, intercepts, etc.) Compare the graph of (x) with the graph of g(x) =/(x - 1). What did you notice? -2 1 2 1 52. Ax) = x a. Graph the function /(x). b. g(x) =f(x + 3) = c. Sketch the Graph of g(x) =/(x + 3) on the same coordinate axis. Be sure to identify which graph is which and label key points (vertices, intercepts, etc.) Compare the graph of /(x) with the graph of g(x) =/(x + 3). What did you notice? -1 -2 -1 1 2 5 In part B, you were adding a constant "c" to the independent variable, or input variable. Can you make a general statement regarding the transformation that occurs when adding of a constant to the dependent variable? What transformation occurs when you add "c" to the input variable of f(x) yielding fixte) ? Explain what happened and why. How does your choice of a value for c affect the basic graph of fix)