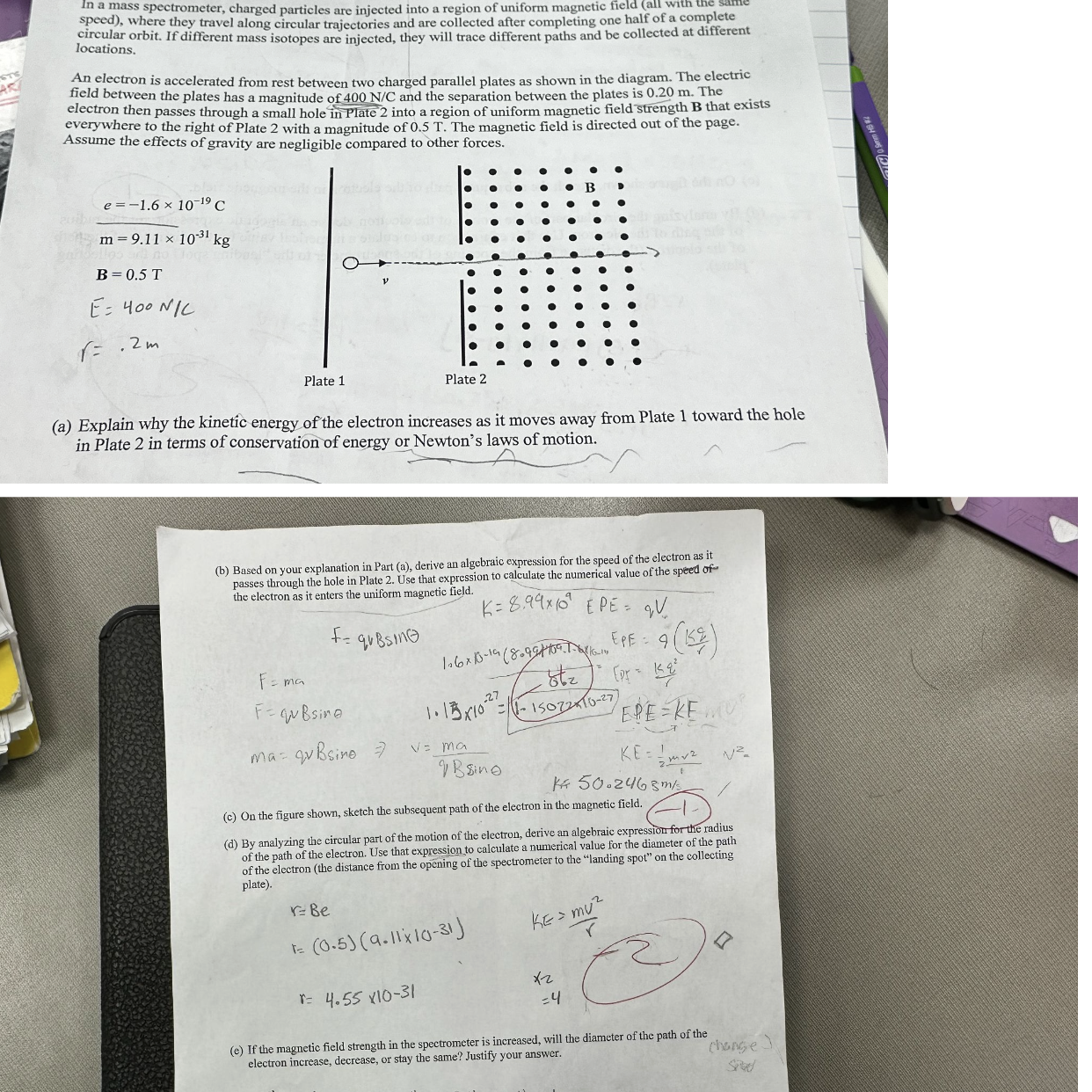
In a mass spectrometer, charged particles are injected into a region of uniform magnetic field (all with the speed), where they travel along circular trajectories and are collected after completing one half of a complete circular orbit. If different mass isotopes are injected, they will trace different paths and be collected at different locations. An electron is accelerated from rest between two charged parallel plates as shown in the diagram. The electric field between the plates has a magnitude of 400 N/C and the separation between the plates is 0.20 m. The electron then passes through a small hole in Plate 2 into a region of uniform magnetic field strength B that exists everywhere to the right of Plate 2 with a magnitude of 0.5 T. The magnetic field is directed out of the page. Assume the effects of gravity are negligible compared to other forces. e=-1.6 x 10-19 C m=9.11 x 1031 kg B=0.5 T . . BD E: 400 NIC f=.2m Plate 1 Plate 2 (a) Explain why the kinetic energy of the electron increases as it moves away from Plate 1 toward the hole in Plate 2 in terms of conservation of energy or Newton's laws of motion. (b) Based on your explanation in Part (a), derive an algebraic expression for the speed of the electron as it passes through the hole in Plate 2. Use that expression to calculate the numerical value of the speed of the electron as it enters the uniform magnetic field. F- qUBsInG F= ma F= quBsine 2V EPE 9 K=8.9910 E PE= 9(158) EPE KE 1.610-19 (80991097-616-1 tz 1.13102-11-1507210-27) ma: qvBsine V= ma Bsino 1 KE=m K 50.2463m/s (c) On the figure shown, sketch the subsequent path of the electron in the magnetic field. (d) By analyzing the circular part of the motion of the electron, derive an algebraic expression for the radius of the path of the electron. Use that expression to calculate a numerical value for the diameter of the path of the electron (the distance from the opening of the spectrometer to the "landing spot" on the collecting plate). r= Be T= (0.5) (9.11x10-31) r= 4.55 x10-31 Kemu x2 =4 (e) If the magnetic field strength in the spectrometer is increased, will the diameter of the path of the electron increase, decrease, or stay the same? Justify your answer. change Spad