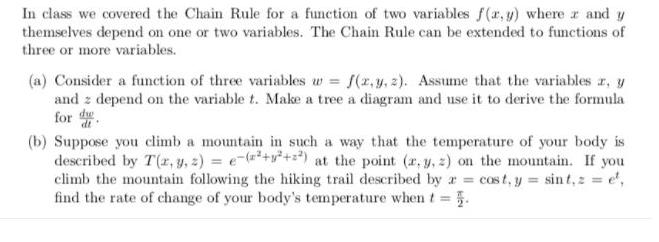
In class we covered the Chain Rule for a function of two variables f(r, y) where r and y themselves depend on one or two variables. The Chain Rule can be extended to functions of three or more variables. (a) Consider a function of three variables w = f(z,y, 2). Assume that the variables z, y and z depend on the variable t. Make a tree a diagram and use it to derive the formula for . (b) Suppose you climb a mountain in such a way that the temperature of your body is described by T(r, y, 2) e-(+v*+=?) at the point (r, y, z) on the mountain. If you climb the mountain following the hiking trail described by a = cost, y = sint, z = e, find the rate of change of your body's temperature when t 5.