Question: if two reference frames, F and F', are moving at con- stant velocity with respect to one another, the acceleration of an object is

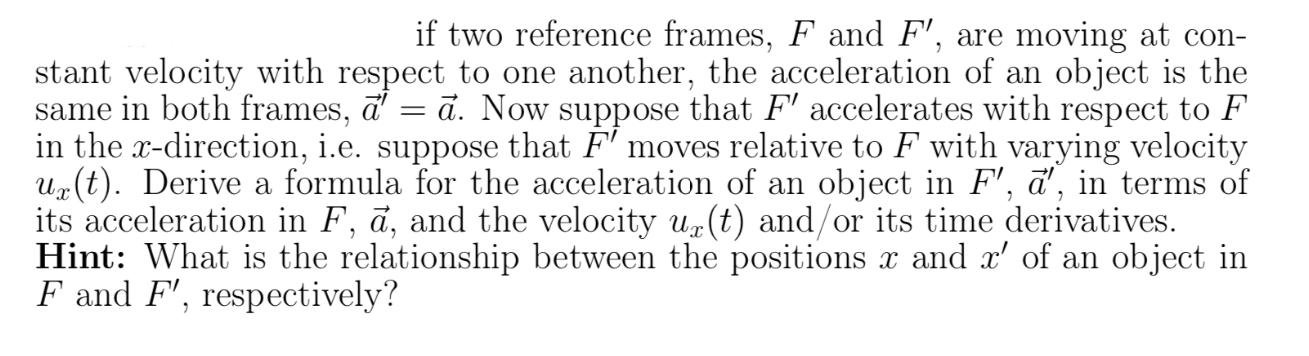
if two reference frames, F and F', are moving at con- stant velocity with respect to one another, the

if two reference frames, F and F', are moving at con- stant velocity with respect to one another, the acceleration of an object is the same in both frames, a' = . Now suppose that F' accelerates with respect to F in the x-direction, i.e. suppose that F' moves relative to F with varying velocity u(t). Derive a formula for the acceleration of an object in F', a', in terms of its acceleration in F, , and the velocity u(t) and/or its time derivatives. Hint: What is the relationship between the positions x and x' of an object in F and F', respectively?

Step by Step Solution

★★★★★

3.57 Rating (157 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

To derive a formula for the acceleration of an object in frame F denoted as a in terms of its accele... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Physics Questions!

Tugela Manufacturing Company (TMC) manufactures heavy duty machinery according to client specifications. On 1 April 2019 the incomplete work consisted of one job no.305, with accumulated cost to an...

Managing Scope Changes Case Study Scope changes on a project can occur regardless of how well the project is planned or executed. Scope changes can be the result of something that was omitted during...

The acceleration of an object moving in the gravitational field of the Earth is a = GMEr/r3 where r is the position vector directed from the center of the Earth toward the object. Choosing the origin...

Need your help Let Us Do Direction: Read each item carefully and encircle the letter of the correct answer. 1. It is the study of space and time and how they relate to each other depending on the...

Answer the following 1. It is defined as the velocity of an object in the rest frame of another object. A. relative motion B. relative velocity C. instantaneous velocity D. instantaneous acceleration...

b. How far has each object travelled at time t = 3 s? c. What is the slope of each line? A 20 g mass and a 50 g mass are dropped from rest from the same height above the oon a. If we ignore air...

(frame 5', particle frame) f ' . fv X _ f s Figure 4.2 (frame S) f f: f). : 7' Figure 4.3 184 Chapter 4. 4evecwrs 4.5 Force and acceleration The goal in this section is to determine how forces and...

Complete the following Carolina Biological Lab: Kinematics. Please read the Distance Learning Lab Safety Agreement. By taking part in these labs, you agree to the terms in the Distance Learning...

Formulas to use to solve the questions below. And use exactly the same formulas as they are in the images below. Same letters and variables in the formulas should be used to solve the problems...

What are the concentrations of HSO42, SO42-, and H+ in a 0.20 M KHSO4 solution?

Consider the series 1. Evaluate the the following limit. If it is infinite, type "infinity" or "inf". If it does not exist, type "DNE". 2. What can you say a bout the series using the Ratio Test?...

1 4 . What does the contribution margin measure? * 3 points A . How much fixed costs a company has B . How much investment an owner has put into a company C . What an owner's salary should be based...

The stock of Quail Corporation is held as follows: 85% by Pheasant Corporation and 15% by Gisela, an individual. Quail Corporation is liquidated in December of the current year pursuant to a plan...

Label any peaks/structures INFRARED SPECTRUM 0.8 0.6 0.4 0.2 2000 1000 3000 Wavenumber (cm-1) Relative Transmittance 1.

Iron has a BCC crystal structure at room temperature, and a lattice constant, a, of 0.286 nm. How many atoms are contained within the (10 0) plane? Answer:

b. Where in nature would you find solutions with more than 1 M Na* and SO dissolved in them? What effect would all that salt have on most dissolution- precipitation reactions?

A3+ has prepared its 3rd quarter budget and provided the following data: The cash balance on June 30 is projected to be $4,000. The company has to maintain a minimum cash balance of $5,000 and is...

Activity-based costing Blank______ be applied to administrative activities.Multiple choice question.cannotcanis required toshould not

To test the effectiveness of protective packaging, a firm shipped out 1200 orders in regular light packaging and 1500 orders in heavy-duty packaging. Of the orders shipped in light packaging, 20...

Following are summary statistics for two data sets, A and B. a. Compute the inter-quartile ranges for both A and B. b. Do the summary statistics for A provide enough information to construct a box...

The number of miles traveled per gallon of gasoline for a certain car has a mean of 25 and a standard deviation of 2. The tank holds 20 gallons. a. Find the mean number of miles traveled per tank. b....

Alexander Corporation (a U.S.-based company) acquired 100 percent of a Swiss company for 8.2 million Swiss francs on December 20, Year 1. At the date of acquisition, the exchange rate was $0.70 per...

How does a parent company determine the appropriate method for translating the financial statements of a foreign subsidiary?

Gramado Company was created as a wholly owned subsidiary of Porto Alegre Corporation on January 1, Year 1. On that date, Porto Alegre invested $42,000 in Gramado's capital stock. Given the exchange...