In equimolar counter diffusion for every mole of one component diffusing in one direction there is$,$ by definition, one mole of the other component diffusing in the opposite direction.

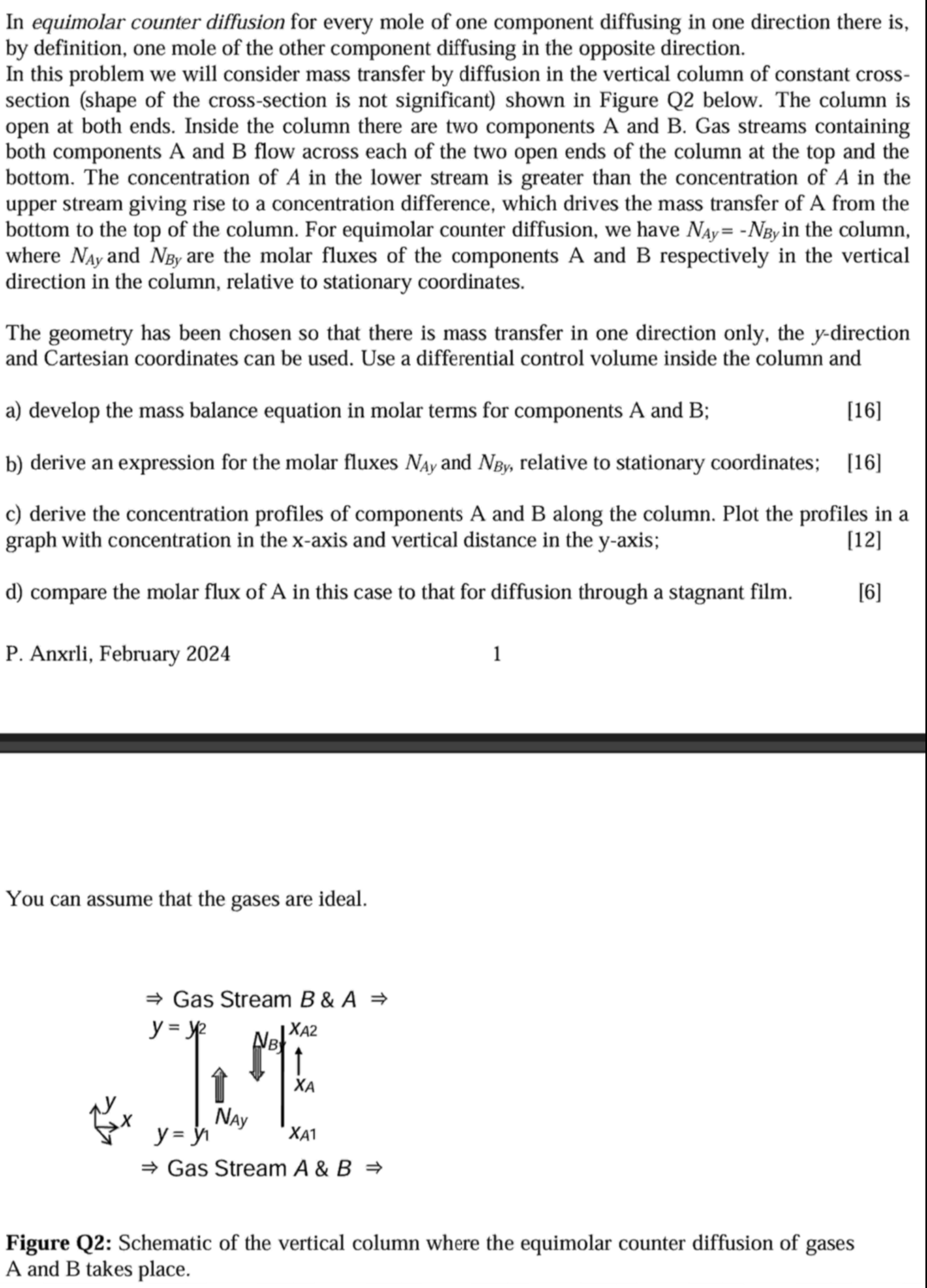
In this problem we will consider mass transfer by diffusion in the vertical column of constant cross$-$ section $($shape of the cross$-$section is not significant$)$ shown in Figure Q$2$ below. The column is open at both ends. Inside the column there are two components A and B$.$ Gas streams containing both components A and B flow across each of the two open ends of the column at the top and the bottom. The concentration of A in the lower stream is greater than the concentration of A in the upper stream giving rise to a concentration difference, which drives the mass transfer of A from the bottom to the top of the column. For equimolar counter diffusion, we have NAy $=-$NBy in the column, where NAy and NBy are the molar fluxes of the components A and B respectively in the vertical direction in the column, relative to stationary coordinates.

The geometry has been chosen so that there is mass transfer in one direction only, the y$-$direction and Cartesian coordinates can be used. Use a differential control volume inside the column and

a$)$ develop the mass balance equation in molar terms for components A and B; $[16]$

b$)$ derive an expression for the molar fluxes NAy and NBy$,$ relative to stationary coordinates; $[16]$

c$)$ derive the concentration profiles of components A and B along the column. Plot the profiles in a graph with concentration in the x$-$axis and vertical distance in the y$-$axis; $[12]$

d$)$ compare the molar flux of A in this case to that for diffusion through a stagnant film.$[6]$

You can assume that the gases are ideal.

$$ Gas Stream B & A $$

y $=$ y$2$

x y $=$ y$1$ NAy

NBy xA$2$

xA xA$1$

$$ Gas Stream A & B $$In equimolar counter diffusion for every mole of one component diffusing in one direction there is$,$

by definition, one mole of the other component diffusing in the opposite direction.

In this problem we will consider mass transfer by diffusion in the vertical column of constant cross$-$

section $($shape of the cross$-$section is not significant$)$ shown in Figure Q$2$ below. The column is

open at both ends. Inside the column there are two components A and B$.$ Gas streams containing

both components A and B flow across each of the two open ends of the column at the top and the

bottom. The concentration of $A$ in the lower stream is greater than the concentration of $A$ in the

upper stream giving rise to a concentration difference, which drives the mass transfer of A from the

bottom to the top of the column. For equimolar counter diffusion, we have $N_{Ay}=-N_{By}$ in the column,

where $N_{Ay}$ and $N_{By}$ are the molar fluxes of the components A and B respectively in the vertical

direction in the column, relative to stationary coordinates.

The geometry has been chosen so that there is mass transfer in one direction only, the $y-$direction

and Cartesian coordinates can be used. Use a differential control volume inside the column and

a$)$ develop the mass balance equation in molar terms for components A and $B$;

b$)$ derive an expression for the molar fluxes $N_{Ay}$ and $N_{By},$ relative to stationary coordinates;

c$)$ derive the concentration profiles of components A and $B$ along the column. Plot the profiles in a

graph with concentration in the $x-$axis and vertical distance in the $y-$axis;

d$)$ compare the molar flux of $A$ in this case to that for diffusion through a stagnant film.

You can assume that the gases are ideal.

Figure Q$2$: Schematic of the vertical column where the equimolar counter diffusion of gases

A and B takes place.