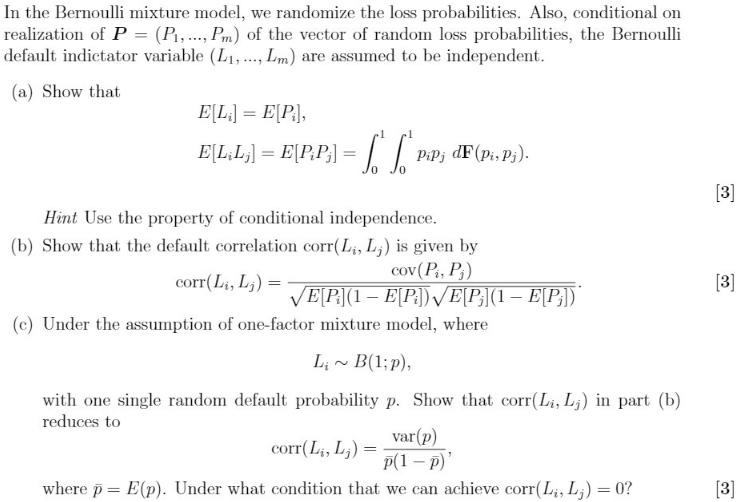
In the Bernoulli mixture model, we randomize the loss probabilities. Also, conditional on realization of P = (P, ..., Pm) of the vector of random loss probabilities, the Bernoulli default indictator variable (L,..., Lm) are assumed to be independent. (a) Show that E[L] = E[P;], E[L;L;] = E[P.P;} = " [" Pipj dF (Pi, Pj). Hint Use the property of conditional independence. (b) Show that the default correlation corr(L, L,) is given by cov (P, P) corr(Li, Lj): E[P](1 - E[P])/E[P;](1 E[P;]) (c) Under the assumption of one-factor mixture model, where L ~ B(1; p), with one single random default probability p. Show that corr(Li, Lj) in part (b) reduces to var(p) p(1-P)' where p = E(p). Under what condition that we can achieve corr(L, Lj) = 0? corr(L, Lj)= = [3] [3] [3]