1. In the caplet example 23.6, construct the synthetic caplet using the three- period zero and mma (the caplet has a strike rate of k 0.04 or 4 percent). Show that the cost of construction is 0.003, the same as that with the two- period zero-coupon bond.

(Attached picture is the example 23.6, the bottom page is the first page)

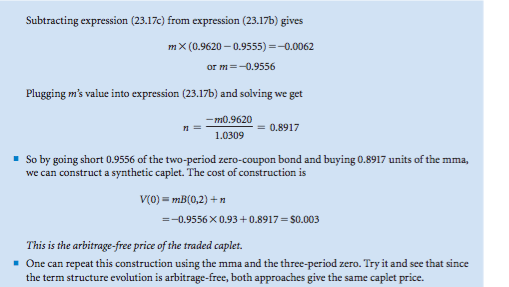
. As in the binomial model of chapter 17 , we construct a portfolio consisting of a zero - coupon bond and mima to replicate the traded caplet's payoffs in both the up and down states . The cost of con - structing this synthetic caplet is the arbitrage - free price of the traded caplet . . Using the zero - coupon bond price evolution in Figure 23. 2 , we can compute the values for the spot rate at time I in the up and down states , R ( 1 ) and R ( 1 ), respectively . R ( 1 ) = 1/ 8 ( 1 , 2 ) - 1 = 1/ ( 0. 9620 ) - 1 = 0.0395 R ( 1 ) = 1/ 8 ( 1 , 2 ) - 1 = 1/ ( 0.9555 ) - 1 = 0.0465 The Caplet's Time 1 Payoffs . Consider the caplet of Figure 23. 4 maturing at time I with strike rate * = 0. 04 per year . In the up state , the caplet ends out - of - the - money with zero value , as the following computation shows :" C = max [ ( R ( 1 ) - K) | ( 1 + R ( 1 ) 1 , 0] = max [ ( 0. 0395- 0.04 ) / 1. 0395, 0] = 0 ( 23. 162) In the down state , the caplet ends up in - the - money with value [ = max [ ( R ( 1 ) - K)| ( 1 + R ( 1 ) ). 0] = max [ ( 0. 0465- 0.04 ) / 1. 0465, 0] = $0. 0062 ( 23. 16 b )FIGURE 23. 4: A Caplet Evolution Time !! Time ! ~# #1) = max / } . ^ - 0.CH` =` Symbols _ # #|I'm = max | 0. 1 + Rilla` ` dilly = max\\O. J . Reilly` Constructing the Synthetic [aplet* . Form a portfolio using in shares of the two- period zero - coupon bond with price Bid, }) and " units of the mima with value Ally . The cast of construction is VID) = MBID, 2 ) + riding |23.17 2) { At time I , we want to find I'm , " ) to match the portfolio's value to the traded caplet's value in each state . "This field's two equations in the unknowns :` In the up state` or ma. SOZO + +1 . 013:04 = 101 123.17 6) In the down state : Vill = mall2\\ THATLY= UL!` or ma. 9555 + +1 . 03:04 = 1. 0.06 2 123.17 0)Subtracting Expression ( 27.17 0 ) from Expression ( 23.170) gives |* 10.9620 - 21.4535) =_ 1.20 0Z UI FM - - 21. 459{ Plugging in's value into Expression ( 13. 17 bl and solving WE Fet |1 = 1. 41305 { babyy going short 1. 4556 of the two _ period zero_ coupon bond and buying U. 6517 units of the IIIma , WIL LET CUTIEtFUEL & Synthetic caplet. The cost of construction 15 - - 1.4556* 01.43 + 1.6917 = 50.0403 This is the arbitrage - free price of the traded raplet { LINE can repeat this construction using the mama and the three - period zero ." IT ; it and see that since the term Structure Evolution is arbitrage _FILE , bath approaches Five the same caplet Price