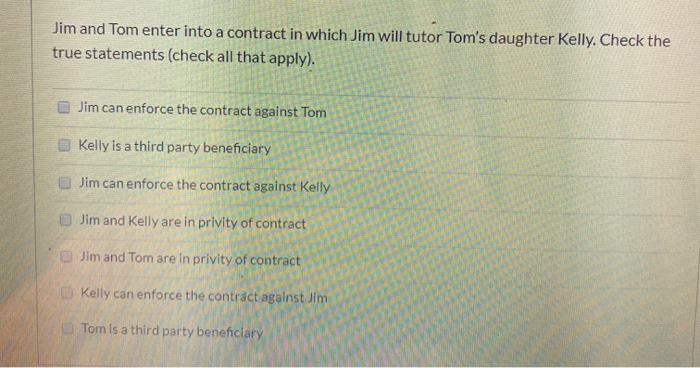
Question
In the Roll model trade prices are pt = mt + cdt where mt is the efficient price (mid-price) and dt = 1 is the
In the Roll model trade prices are pt = mt + cdt where mt is the efficient price (mid-price) and dt = 1 is the trade sign (indicator) which shows if the trade is a buy or sell.
The simple Roll model assumes that trade signs are serially uncorrelated: corr(dt, ds) = 0 for t ?= s. In practice, trade signs are correlated, with positive autocorrelation. Suppose that corr(dt, dt?1) = ? > 0 and corr(dt, dt?k) = 0 for k > 1. Suppose that ? > 0 is known. Also, assume that the trade signs dt are uncorrelated with the increments of the efficient price ?mt = ut.
i) Show that V ar(?pt) = 2c2(1 ? ?) + ?u2, Cov(?pt, ?pt?1) = ?c2(1 ? 2?), Cov(?pt, ?pt?2) = ?c2?, and Cov(?pt, ?pt?k) = 0 for k > 2.
ii) Suppose that 0
Hints. For (i), recall the corresponding expressions in the simple Roll 222
model V ar(?pt) = 2c + ?u and Cov(?pt, ?pt?1) = ?c .
Note that the equations above in (i) reduce to these expressions if ? = 0. Repeat the computations for the simple model allowing for the correlation ? and you should get the results stated.



Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


