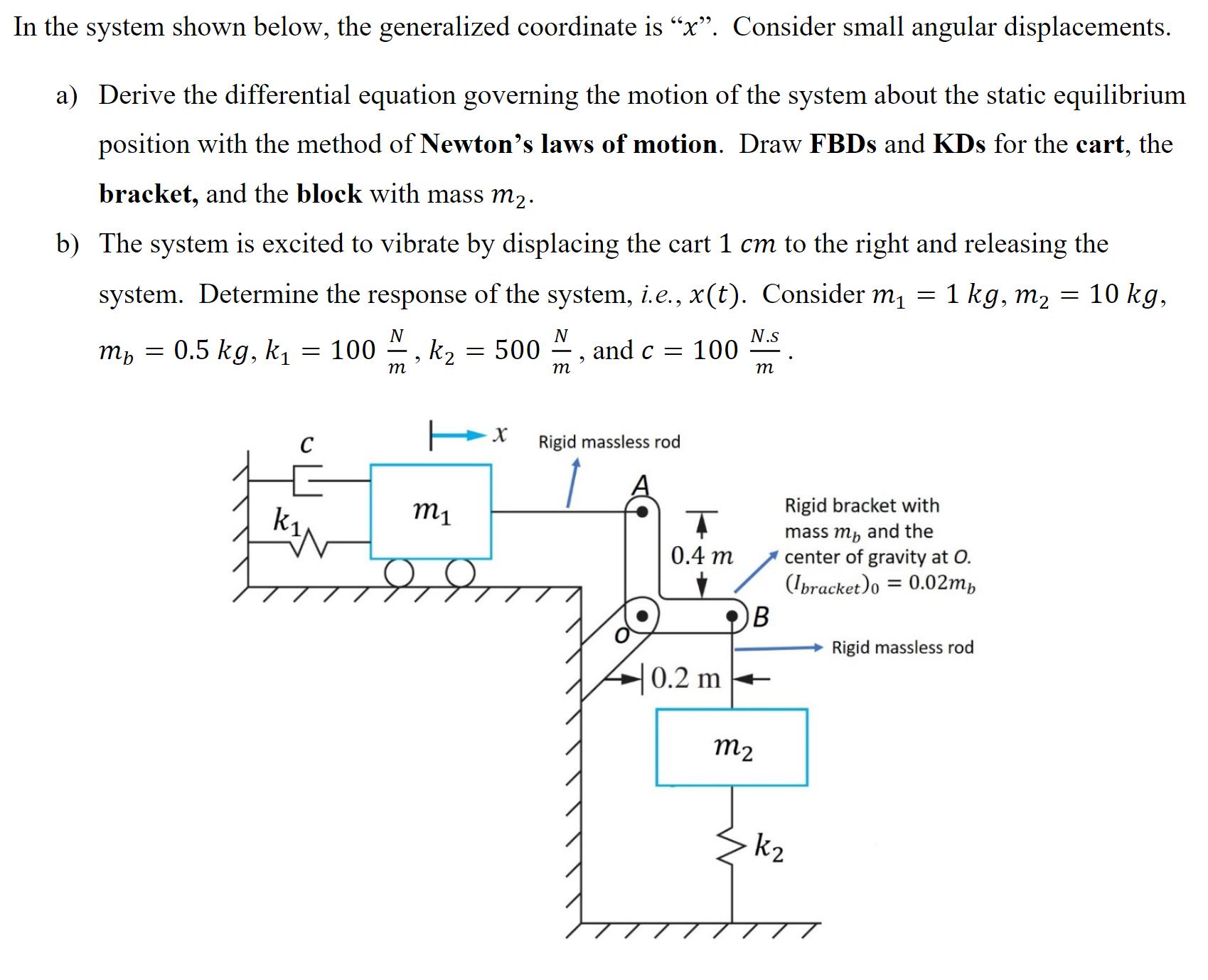
In the system shown below, the generalized coordinate is "x". Consider small angular displacements. a) Derive the differential equation governing the motion of the system about the static equilibrium position with the method of Newton's laws of motion. Draw FBDS and KDs for the cart, the bracket, and the block with mass m2. b) The system is excited to vibrate by displacing the cart 1 cm to the right and releasing the system. Determine the response of the system, i.e., x(t). Consider m1 = 1 kg, m2 = 10 kg, N.s and c = 100 N m, = 0.5 kg, k, = 100 " , k, 500 m m m C Rigid massless rod Rigid bracket with mass m, and the center of gravity at O. (Ibracket)o = 0.02m, k m1 0.4 m B Rigid massless rod |0.2 m + m2 k2