In this exercise you will be following the methods of the Ancients (Greek Astronomers of Alexandria) to determine the size of the Earth. Most of these measurements were quite difficult for the ancient astronomers to carry out and although you will be following their methods you will be using better observations than were available to them. In fact, you will see that the simple model which the Ancients had of the Sun-Earth-Moon system allows quite accurate determination of distances when good observations are used.

Directions: The size of the Earth

This step was first performed by Eratosthenes (276-196 BC) and involves a very simple model for just the Sun and the Earth, it is:

1. The Earth is a sphere.

2. The Sun is very far away.

Eratosthenes knew that at noon on June 21 (the summer solstice - longest day), the Sun would shine into the bottom of a deep vertical pit in the city of Syene, meaning the Sun was at the zenith in the sky at this time. Being in Alexandria, which was located more or less due north of Syene, he could measure the length of a shadow cast by an obelisk at the same time, noon on the summer solstice.

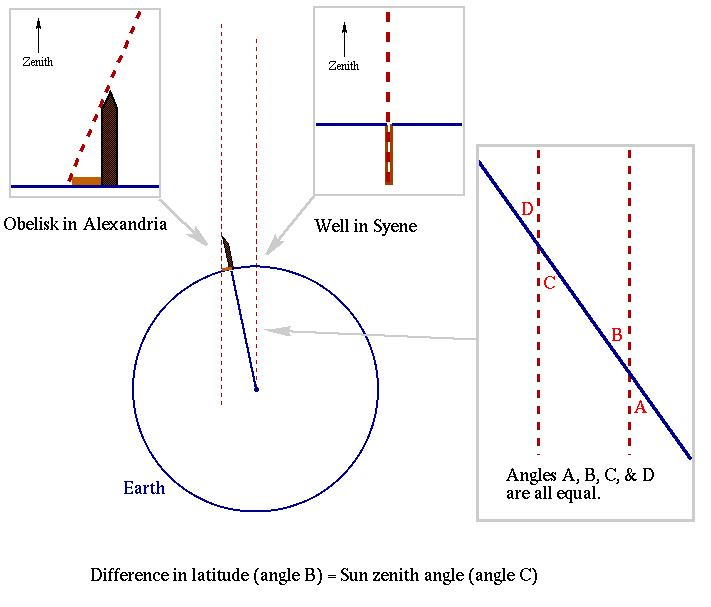
This measurement, along with the height of the obelisk, gave him the angle that the Sun appeared south of the zenith in Alexandria, an angle he determined to be 7.2 degrees. The Sun was not as high in the sky in Alexandria as in Syene at the same time of day and the same time of year.

This is in fact a measurement of the curvature of the Earth's sphere between Syene and Alexandria, and once distance of this small arc is known, the circumference of the entire Earth is determined. To this end (astronomers having considerably more political clout in those days!) he ordered some soldiers to march off the distance between Alexandria and Syene, a distance of 5000 stadia (thought today to be equivalent to about 500 miles).

The circumference of the Earth could be found by using the following proportionality:

Syene to Alexandria                    7.2 degrees

-----------------------             =         ---------

circumference of the Earth            360 degrees

Equation 1

Next Step: Estimating the size of our measuring sticks.   Please follow the directions below to make your own measurements. Feel free to start over and walk through this a couple of times. Basically, you are going to figure out the size of an object using the size of people next to them as a measuring stick. Once you know the size of the object and its shadow, you can use this to fill in Equation 2 below.

Using a similar triangles construction like the one shown in the above figure, we can directly determine the radius of the Earth. The length of the shadow is to the distance between Syene and Alexandria as the height of the obelisk is to the radius of the Earth.   To measure from this picture, we are going to have to get a ruler and do some simple math.

1.     First, we need a scaling factor – our measured distance and the distance in reality.

1.     Let’s assume he is an average height for the early 19^th Century – about 5’ 6” = 66 inches or 1.68 meters.
2.     Using your ruler, how tall is he (in inches or centimeters)?
3.      Divide his real height by your measured height – this is our scaling factor (meters/centimeter or inches/inch, depending on your ruler).

2.     Measure the shadow from the right bottom corner of the obelisk to where it ends (just to the left of the person you just measured).
3.     Measure the height of the obelisk from the base to the very tip.
4.     Now, convert your ruler measurements from #2 and #3 using the scaling factor.
5.     Those values for the shadow and the height get plugged into the equation below.

We can write this in equation form as:

Syene to Alexandria        Length of the Shadow

-------------------     =     --------------------

Radius of the Earth         Height of the Obelisk

Equation 2

Part 2 Questions: Answer the ones below.

• For this experiment we assume that the Sun is very far away. What is the geometric significance of assuming the Sun to be very far away for geocentric theory?
• What value for the circumference of the Earth would Eratosthenes have determined from his measurements? (Convert your answer to kilometers – converting to meters here)
• What value for the radius of the Earth did he determine? (You can use the formula for the circumference of a circle.)
• Using Equation 2 and your measurements from the sketch, calculate the radius of the Earth in kilometers.
• What would be your sources of error here (since Question 8 does not equal Question 9)? Discuss three.

P.S TEACHER IS ASKING ME TO USE THE TOWER TO MAKE MY MEASUREMENT.
 

Zenith Obelisk in Alexandria Earth Zenith Well in Syene B Difference in latitude (angle B) = Sun zenith angle (angle C) A Angles A, B, C, & D are all equal.