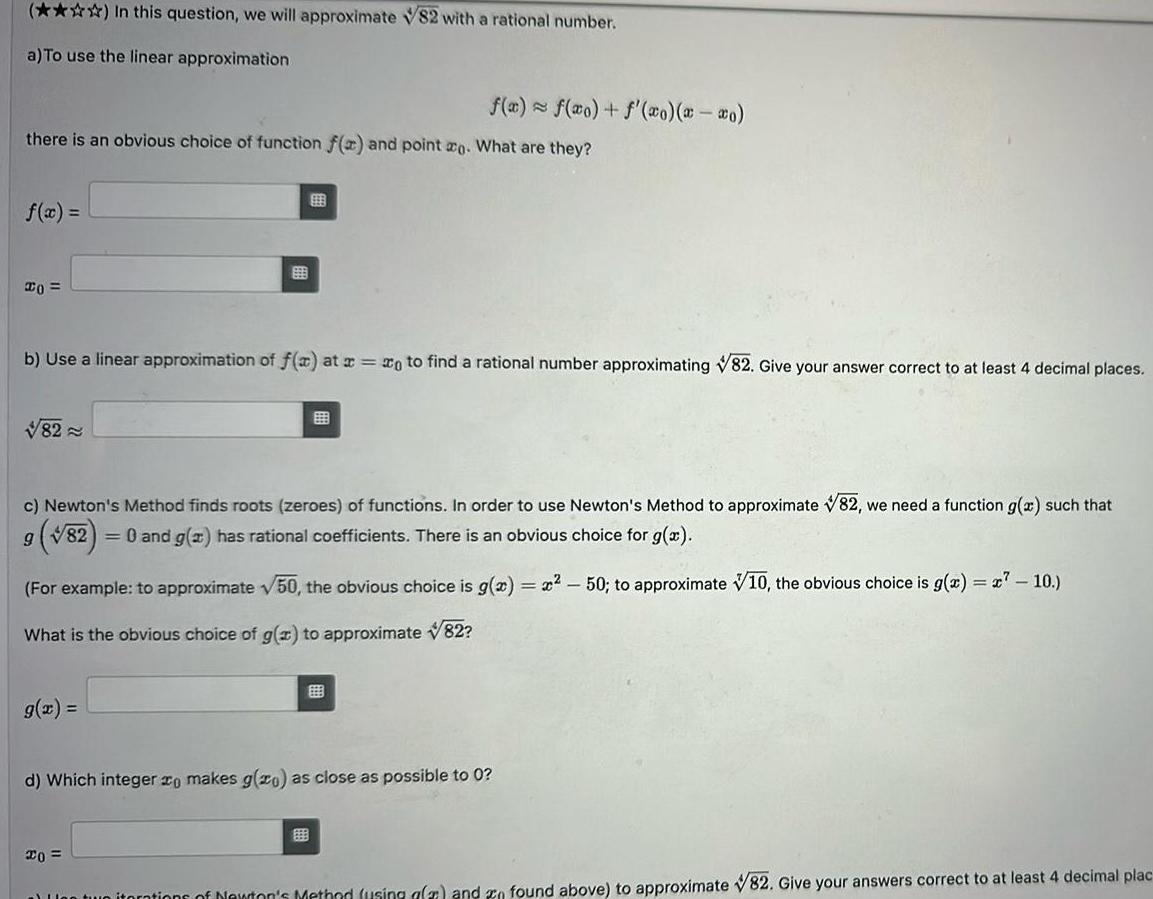
() In this question, we will approximate 82 with a rational number. a) To use the linear approximation f(a) f(o)+f'(20)(-20) there is an obvious choice of function f(x) and point o. What are they? f(x) = =0x b) Use a linear approximation of f(a) at x=xg to find a rational number approximating 82. Give your answer correct to at least 4 decimal places. 82 c) Newton's Method finds roots (zeroes) of functions. In order to use Newton's Method to approximate 82, we need a function g(x) such that g(82) = 0 and g(x) has rational coefficients. There is an obvious choice for g(x). (For example: to approximate 50, the obvious choice is g(x) = x - 50; to approximate 10, the obvious choice is g(x) = x7 - 10.) What is the obvious choice of g(x) to approximate 82? 9(x) = d) Which integer 20 makes g(20) as close as possible to 0? 20= Hee two iterations of Newton's Method (using a(z) and 2 found above) to approximate 82. Give your answers correct to at least 4 decimal plac