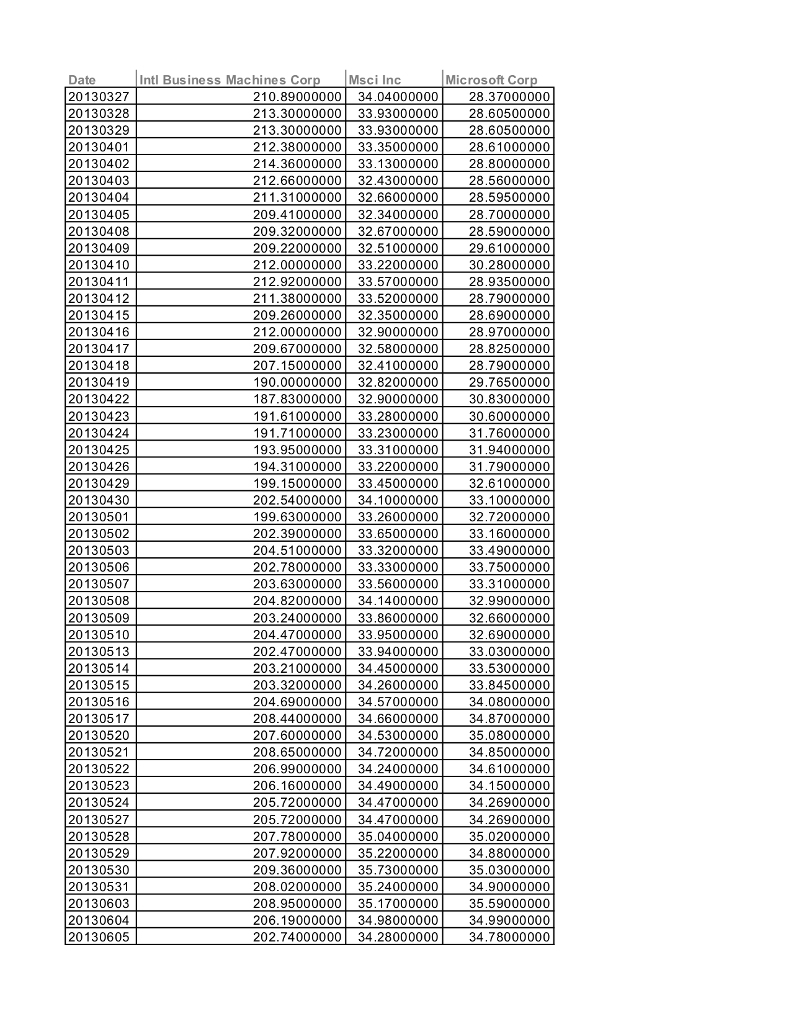
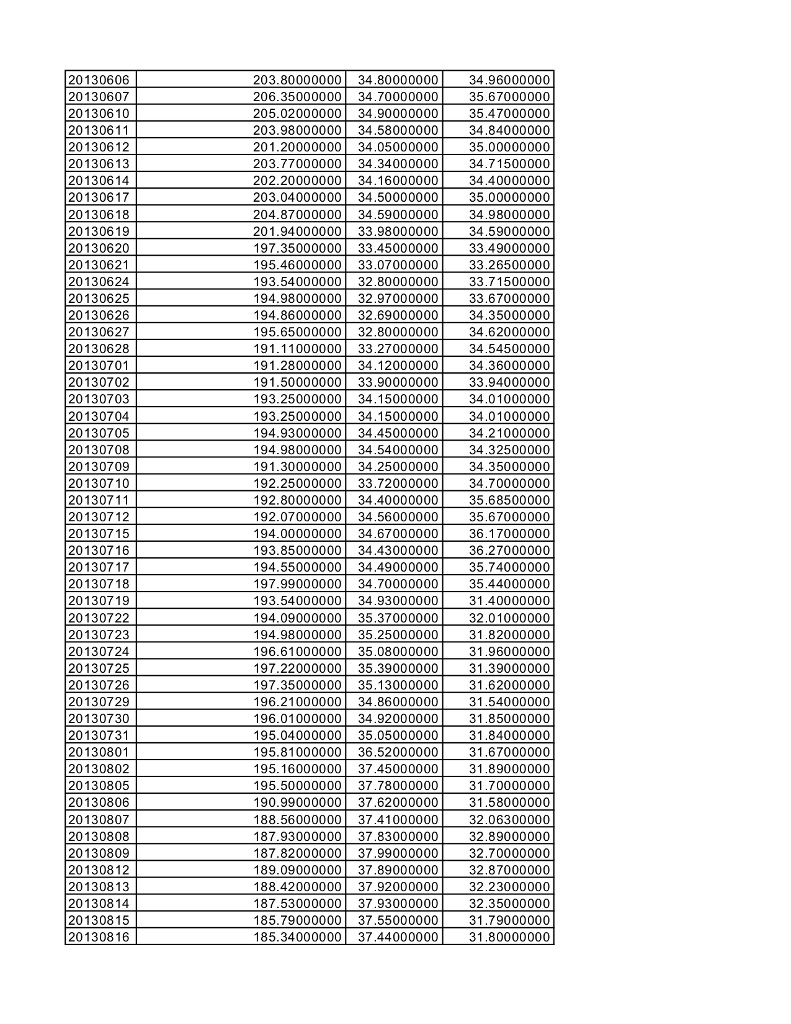
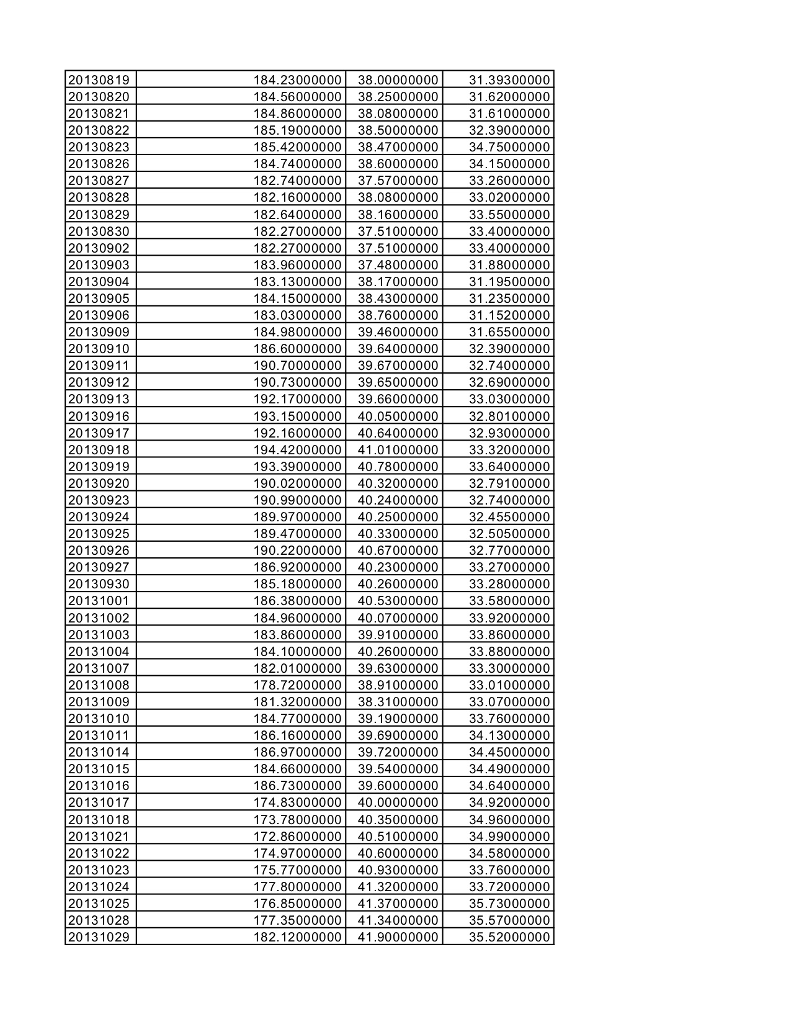
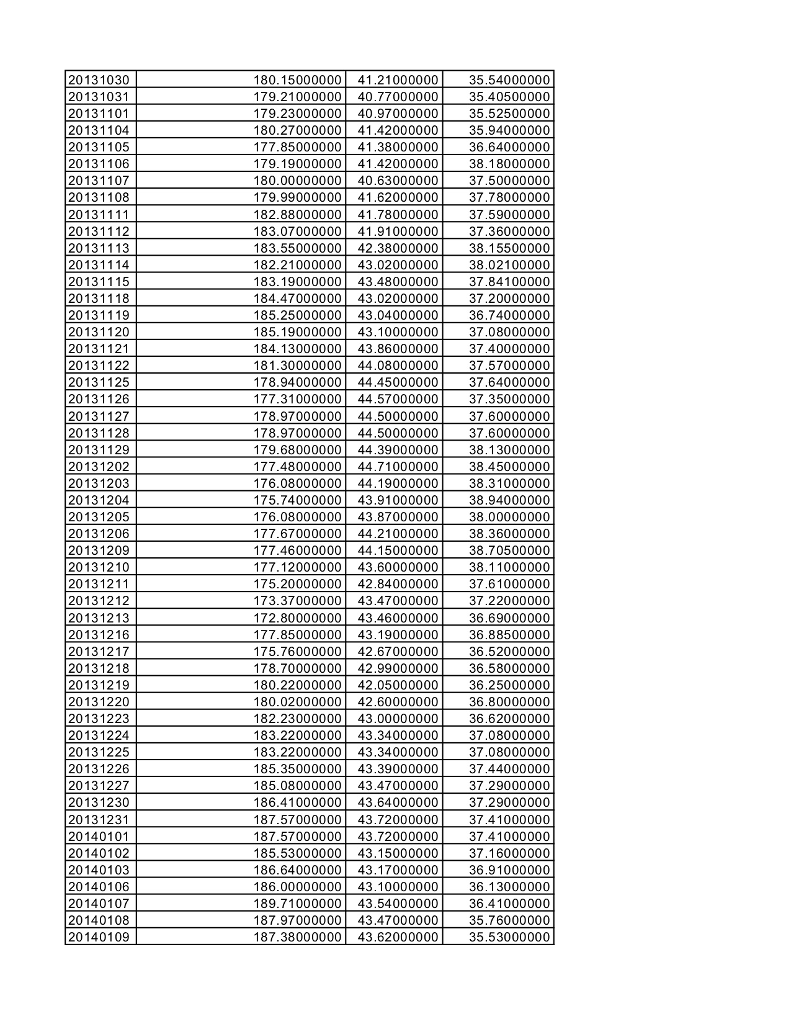
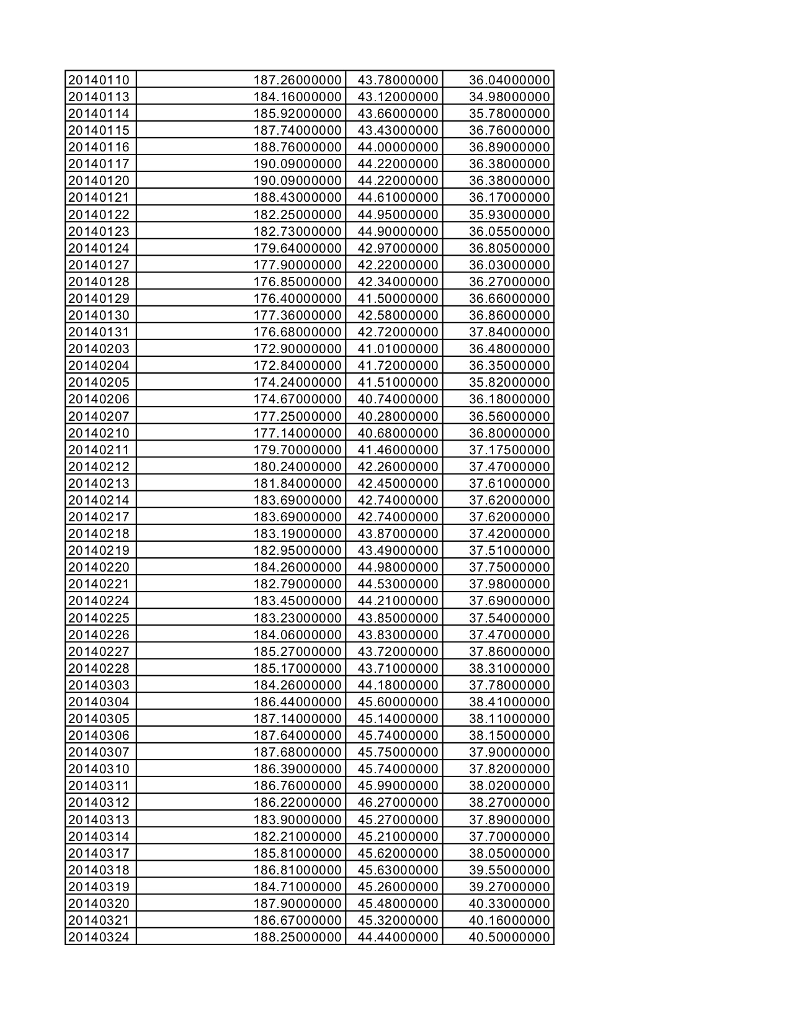
Interest Rate Swan Let T, 1-1, ,n be a set of dates, on which payments of the floating leg of an interest rate swap occur. The payoff of the floating leg of the swap at time Ti is Fi +s where Fi is the reference rate of the floating leg and s is a constant spread. For simplicity, let's assume that the floating and fixed payments happen on the same dates. Also, r is the risk-free rate on the same tenor. Let N be the notional of the swap 1) What is the fixed semiannual swap rate calculated from the risk-free rates? Please specify mathematical formula (no need for exact numerical result at this point) 2) Let the semiannual swap rate calculated in 1) be the fixed leg payment of the swap. What is the constant spread s which sets the present value of the swap position to be zero? Please specify mathematical formula (no need for exact numerical result at this point). The risk free curve and reference curve data can be found in the Excel file. Let's suppose the analysis date is 12 June 2013 and both fixed and floating payment happens semi- annually, with the first payment date half year from analysis date. The swap notional equals to USD 100 000, the maturity of the swap is 5 years, and the swap currency is USD Please provide a MATLAB code or Excel spreadsheet that computes 1) The answer for the first two questions based on the input data given in the attached Excel file 2) The PV of the swap position on 12 August 2013 3) The PV of the swap position on 12 February 2014 You can write down the code with any programming language you are familiar with, and summary of the results should be given in a PDF document. The codes/spreadsheets used for the calculations should be sent in a zipped file together with the PDF document Interest Rate Swan Let T, 1-1, ,n be a set of dates, on which payments of the floating leg of an interest rate swap occur. The payoff of the floating leg of the swap at time Ti is Fi +s where Fi is the reference rate of the floating leg and s is a constant spread. For simplicity, let's assume that the floating and fixed payments happen on the same dates. Also, r is the risk-free rate on the same tenor. Let N be the notional of the swap 1) What is the fixed semiannual swap rate calculated from the risk-free rates? Please specify mathematical formula (no need for exact numerical result at this point) 2) Let the semiannual swap rate calculated in 1) be the fixed leg payment of the swap. What is the constant spread s which sets the present value of the swap position to be zero? Please specify mathematical formula (no need for exact numerical result at this point). The risk free curve and reference curve data can be found in the Excel file. Let's suppose the analysis date is 12 June 2013 and both fixed and floating payment happens semi- annually, with the first payment date half year from analysis date. The swap notional equals to USD 100 000, the maturity of the swap is 5 years, and the swap currency is USD Please provide a MATLAB code or Excel spreadsheet that computes 1) The answer for the first two questions based on the input data given in the attached Excel file 2) The PV of the swap position on 12 August 2013 3) The PV of the swap position on 12 February 2014 You can write down the code with any programming language you are familiar with, and summary of the results should be given in a PDF document. The codes/spreadsheets used for the calculations should be sent in a zipped file together with the PDF document