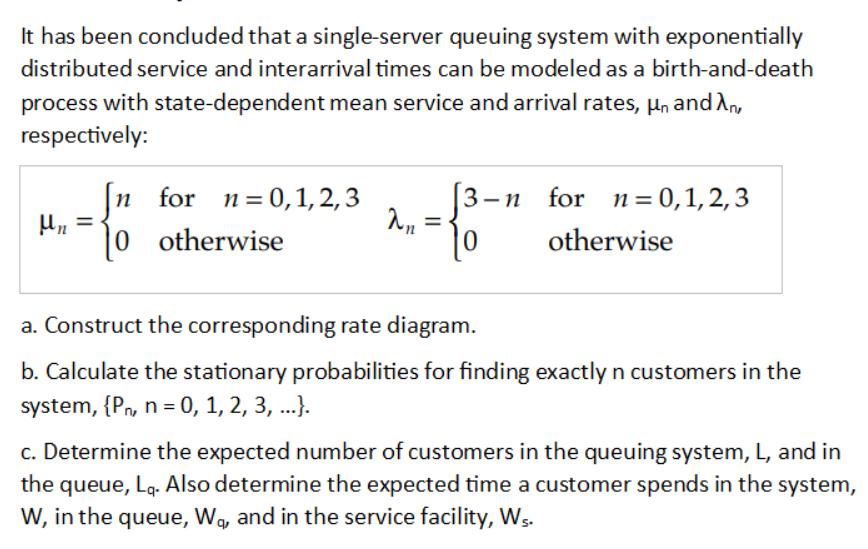
It has been concluded that a single-server queuing system with exponentially distributed service and interarrival times can be modeled as a birth-and-death process with state-dependent mean service and arrival rates, n and n, respectively: = n1 for n= 0, 1, 2, 3 n 0 otherwise = 'n 3-n for n=0,1,2,3 otherwise a. Construct the corresponding rate diagram. b. Calculate the stationary probabilities for finding exactly n customers in the system, {Pn, n = 0, 1, 2, 3, ...}. c. Determine the expected number of customers in the queuing system, L, and in the queue, Lq. Also determine the expected time a customer spends in the system, W, in the queue, Wa, and in the service facility, Ws.