It is required to design an optimum receiver for a digital communication system. Assume that the channel has noise w(t) which is AWGN with PSD of N₀/2. The pulse-shaping filter g(t) is defined as

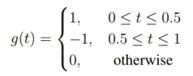
The receiver filter has the following impulse response, instead of the optimum filter:

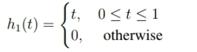
a) Find the output of the receiver filter g_o(t) i.e. g(t) * h₁ (t).

b) Assuming the absence of AWGN, plot the output of the receiver, after the sampler, if the polar input signal is {1,-1,1}.

Transcribed Image Text:

1, 0t 0.5 g(t) = ( -1,0.5 < t < 1 t1 0, otherwise 1, 0t 0.5 g(t) = ( -1,0.5 < t < 1 t1 0, otherwise

Answer rating: 100% (QA)

a Receiver filter output got gt ht Convolution in the time domain is equivalent to multiplication in ... View the full answer