It's about the Arbitrage Theorem, please solve it step-by-step.

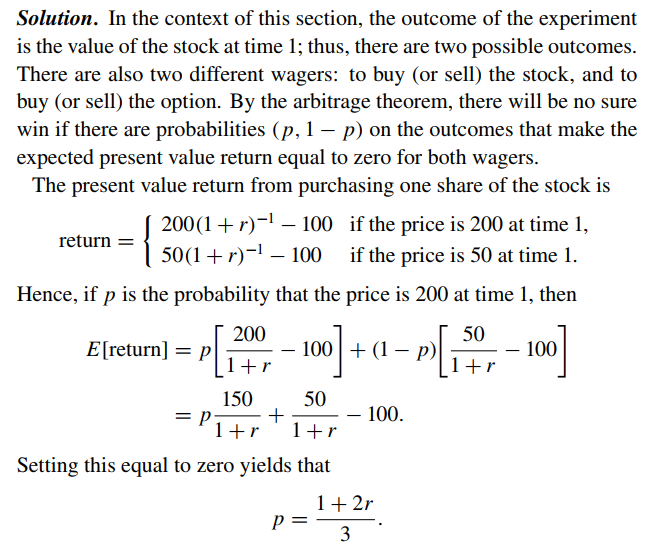
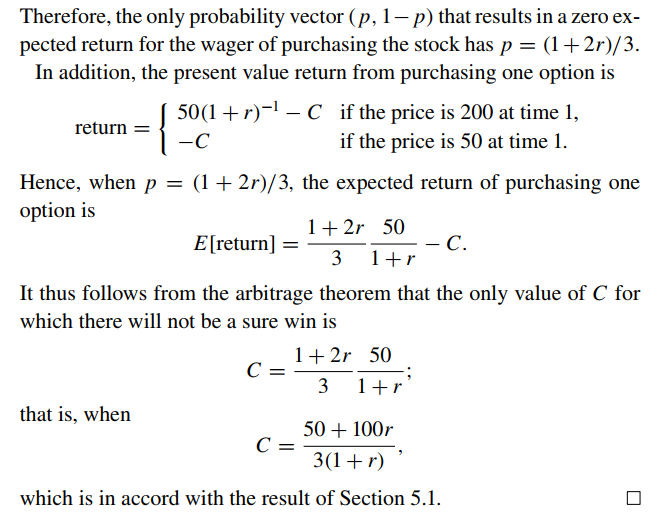
Exercise 6.6 In Example 6.1b, suppose one also has the option of pur- chasing a put option that allows its holder to put the stock for sale at the end of one period for a price of 150. Determine the value of P, the cost of the put, if there is to be no arbitrage; then show that the resulting call and put prices satisfy the put-call option parity formula (Proposition 5.2.2). Example 6.1b Let us reconsider the option pricing example of Sec- tion 5.1, where the initial price of a stock is 100 and the price after one period is assumed to be either 200 or 50. At a cost of C per share, we can purchase at time 0 the option to buy the stock at time 1 for the price of 150. For what value of C is no sure win possible? Solution. In the context of this section, the outcome of the experiment is the value of the stock at time 1; thus, there are two possible outcomes. There are also two different wagers: to buy (or sell) the stock, and to buy (or sell) the option. By the arbitrage theorem, there will be no sure win if there are probabilities (p, 1 p) on the outcomes that make the expected present value return equal to zero for both wagers. The present value return from purchasing one share of the stock is 200(1+r)-! 100 if the price is 200 at time 1, return = 50(1+r)-1 100 if the price is 50 at time 1. Hence, if p is the probability that the price is 200 at time 1, then 50 E[return] =p [ 290 100] +0 P[1. 100 ] +r 1+ 150 50 + 1+r 1+r =P 100. Setting this equal to zero yields that 1+ 2r p= 3 Therefore, the only probability vector (p, 1-p) that results in a zero ex- pected return for the wager of purchasing the stock has p = (1+2r)/3. In addition, the present value return from purchasing one option is 50(1+r)-1-C if the price is 200 at time 1, return = -C if the price is 50 at time 1. Hence, when p = (1 + 2r)/3, the expected return of purchasing one option is 1+ 2r 50 E[return] = -C. 3 . 1+r It thus follows from the arbitrage theorem that the only value of C for which there will not be a sure win is 1+ 2r 50 C= 3 1+r ) that is, when 50 + 100r C= 3(1+r) which is in accord with the result of Section 5.1