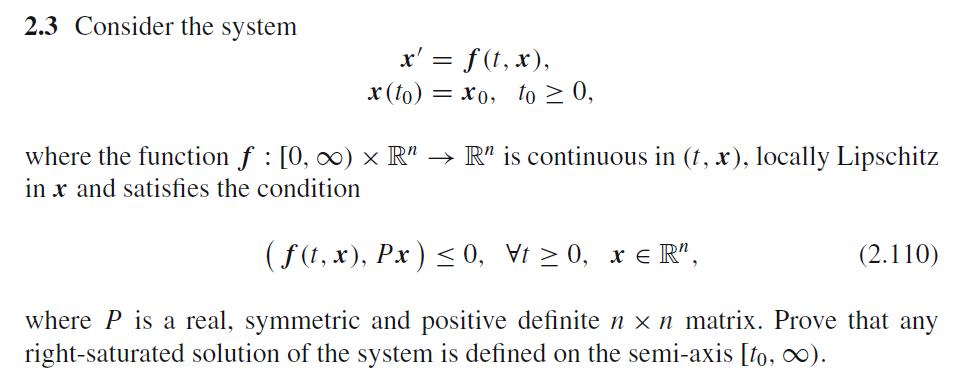
2.3 Consider the system x' = f(t, x), x (to) = xo, to 0, where the function f : [0, ) x R" R" is continuous in (t, x), locally Lipschitz in x and satisfies the condition (f(t, x), Px) 0, \t0, x R", where P is a real, symmetric and positive definite n x n matrix. Prove that any right-saturated solution of the system is defined on the semi-axis [to, ). (2.110)