Question
Jack has an unlimited number of ornamental tiles each comprised of three unit squares. Some tiles are straight 31 tiles, which come in 2 different
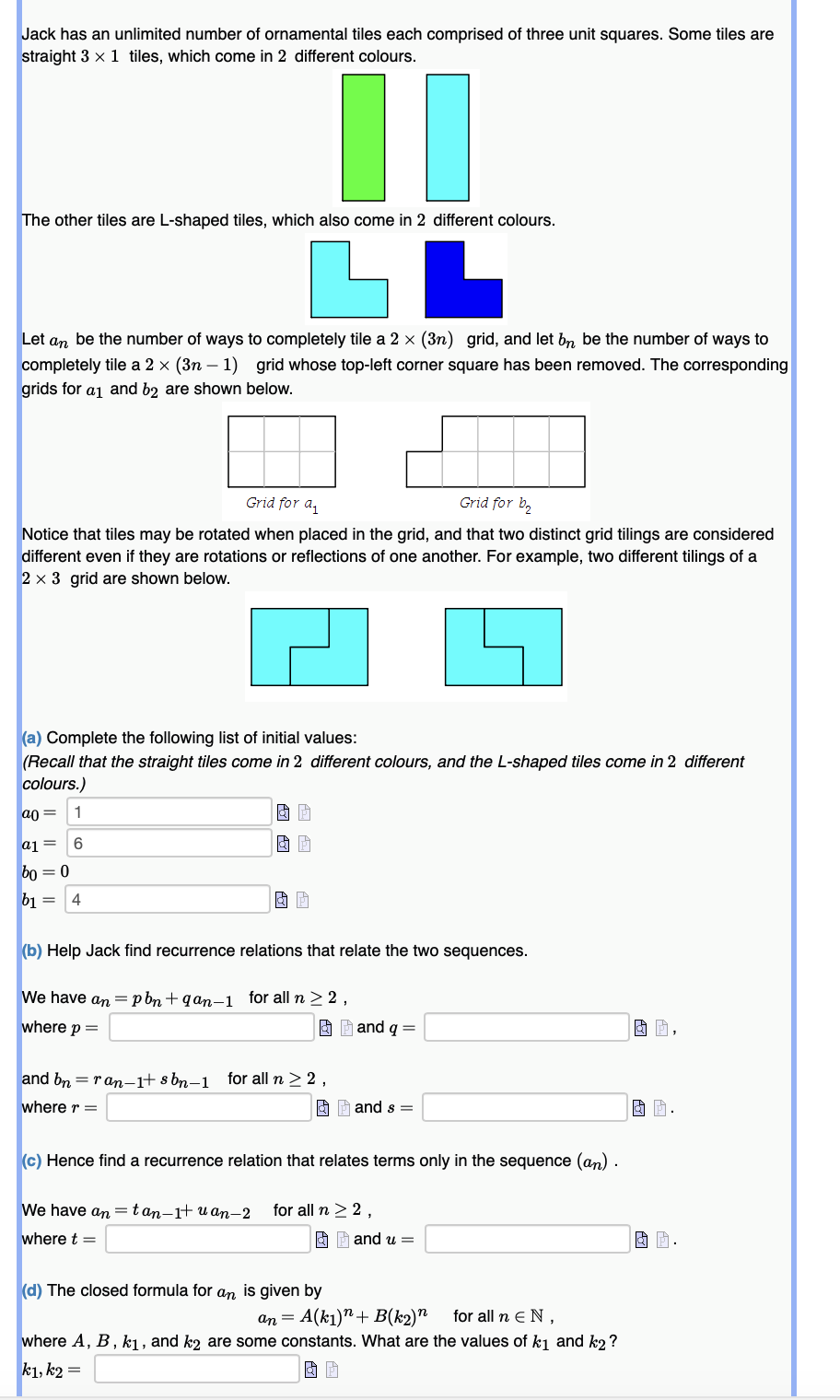 Jack has an unlimited number of ornamental tiles each comprised of three unit squares. Some tiles are straight 31 tiles, which come in 2 different colours. Maple plot The other tiles are L-shaped tiles, which also come in 2 different colours. Maple plot Let an be the number of ways to completely tile a 2(3n) grid, and let bn be the number of ways to completely tile a 2(3n1) grid whose top-left corner square has been removed. The corresponding grids for a1 and b2 are shown below. Maple plot Notice that tiles may be rotated when placed in the grid, and that two
Jack has an unlimited number of ornamental tiles each comprised of three unit squares. Some tiles are straight 31 tiles, which come in 2 different colours. Maple plot The other tiles are L-shaped tiles, which also come in 2 different colours. Maple plot Let an be the number of ways to completely tile a 2(3n) grid, and let bn be the number of ways to completely tile a 2(3n1) grid whose top-left corner square has been removed. The corresponding grids for a1 and b2 are shown below. Maple plot Notice that tiles may be rotated when placed in the grid, and that two
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


