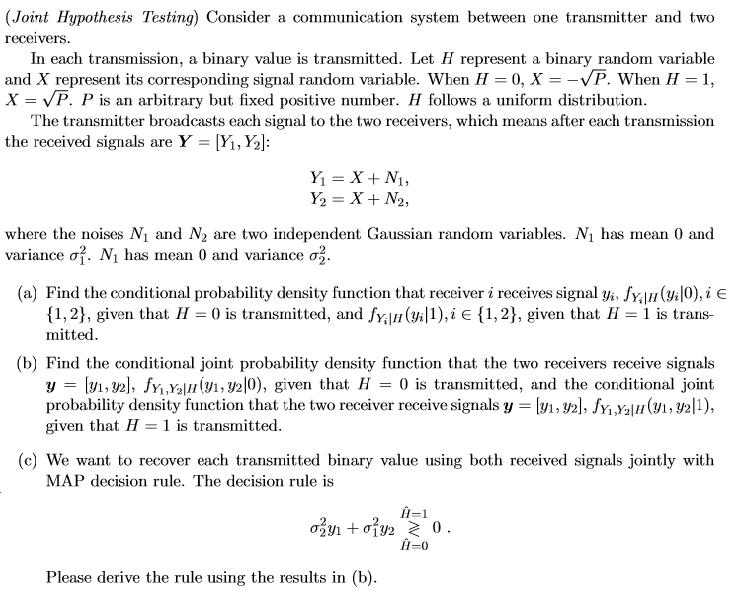
(Joint Hypothesis Testing) Consider a communication system between one transmitter and two receivers. In each transmission, a binary value is transmitted. Let H represent a binary random variable and X represent its corresponding signal random variable. When H = 0, X = P. When H = 1, X = P. P is an arbitrary but fixed positive number. H follows a uniform distribution. The transmitter broadcasts each signal to the two receivers, which means after each transmission the received signals are Y = [Y1, Y2]: Y = X + N1, Y = X + N2, where the noises N and N are two independent Gaussian random variables. N has mean 0 and variance . N has mean 0 and variance . (a) Find the conditional probability density function that receiver i receives signal y, fy|H (yi|0), i {1,2}, given that H = 0 is transmitted, and fy (1), i E {1,2}, given that H = 1 is trans- mitted. (b) Find the conditional joint probability density function that the two receivers receive signals y = [1,2], fyH (31, 32|0), given that H = 0 is transmitted, and the conditional joint probability density function that the two receiver receive signals y = [1, 2], fy,Y2|H (Y1, y2|1), given that H1 is transmitted. (c) We want to recover each transmitted binary value using both received signals jointly with MAP decision rule. The decision rule is =1 +0. Please derive the rule using the results in (b).