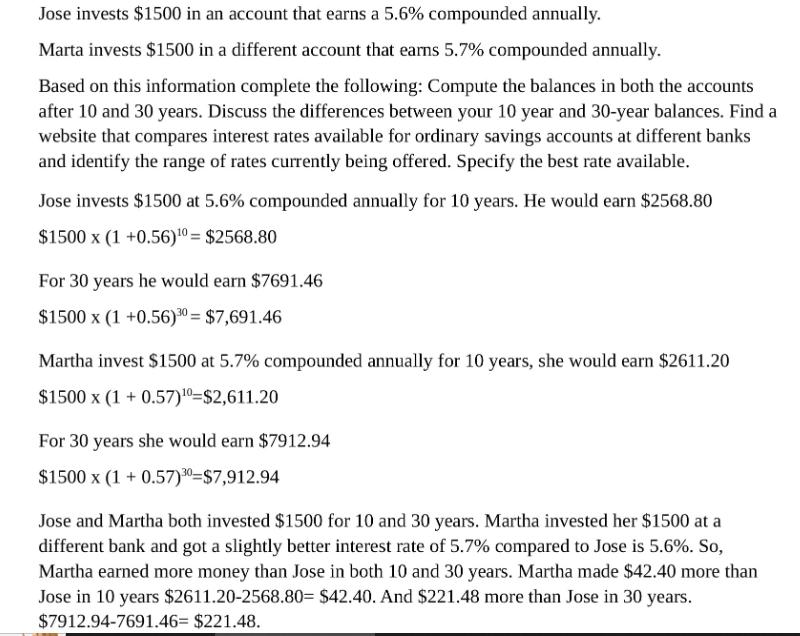
Jose invests $1500 in an account that earns a 5.6% compounded annually. Marta invests $1500 in a different account that earns 5.7% compounded annually. Based on this information complete the following: Compute the balances in both the accounts after 10 and 30 years. Discuss the differences between your 10 year and 30-year balances. Find a website that compares interest rates available for ordinary savings accounts at different banks and identify the range of rates currently being offered. Specify the best rate available. Jose invests $1500 at 5.6% compounded annually for 10 years. He would earn $2568.80 $1500 x (1 +0.56) 10 = $2568.80 For 30 years he would earn $7691.46 $1500 x (1 +0.56) 30 = $7,691.46 Martha invest $1500 at 5.7% compounded annually for 10 years, she would earn $2611.20 $1500 x (1+0.57) 10=$2,611.20 For 30 years she would earn $7912.94 $1500 x (1+0.57) 30-$7,912.94 Jose and Martha both invested $1500 for 10 and 30 years. Martha invested her $1500 at a different bank and got a slightly better interest rate of 5.7% compared to Jose is 5.6%. So, Martha earned more money than Jose in both 10 and 30 years. Martha made $42.40 more than Jose in 10 years $2611.20-2568.80= $42.40. And $221.48 more than Jose in 30 years. $7912.94-7691.46= $221.48.