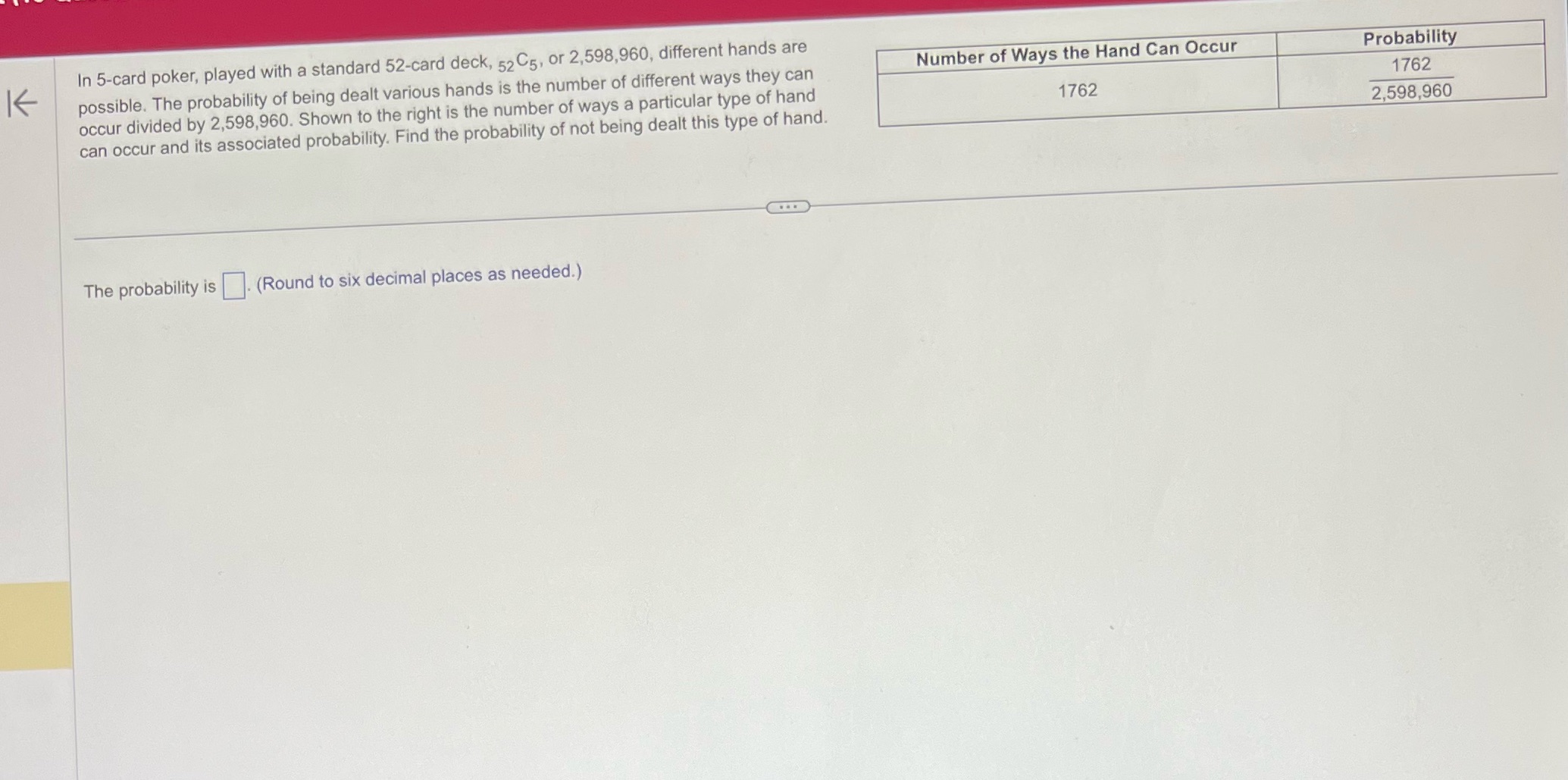
K In 5-card poker, played with a standard 52-card deck, 52 C5, or 2,598,960, different hands are possible. The probability of being dealt various hands is the number of different ways they can occur divided by 2,598,960. Shown to the right is the number of ways a particular type of hand can occur and its associated probability. Find the probability of not being dealt this type of hand. Number of Ways the Hand Can Occur 1762 The probability is (Round to six decimal places as needed.) Probability 1762 2,598,960