
Kindly help tutor
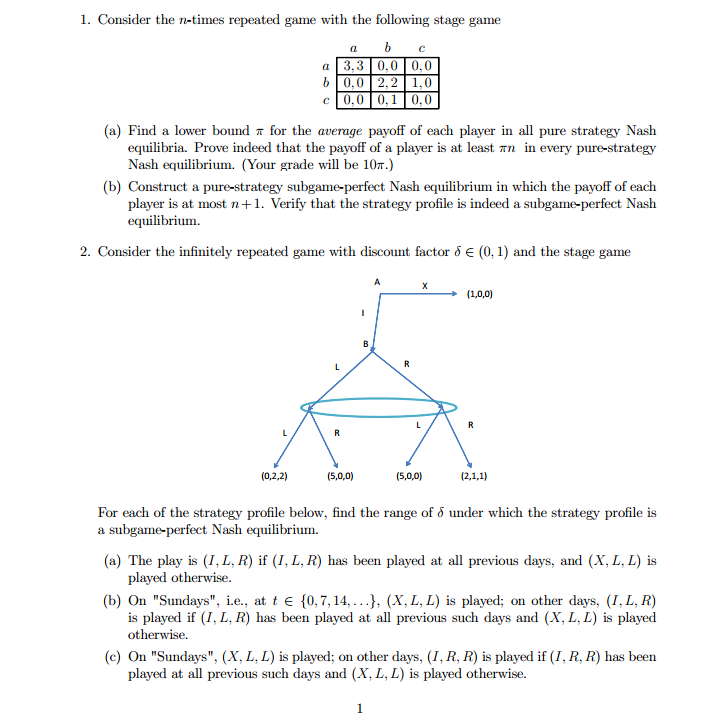
Consider the following standard Homer Model and a variation that includes human capital. Goods Production Flmction Ideas Production Function Human Capital Production Function Resource Constraint Allocation of Labour Human Capital Y. = as... 15AM] : EAthLGJ H: 2 L511; Lyt+La3g +LM = L La; : TE Assume parameters take the following values in each specication. Standard Human lCapital E 1000 1000 Ly; 750 700 LG; 250 200 La; r 100 :2 0.0005 0.0005 A5 10 1 l] 1. Explain what using labour to produce human capital implies for the economy? 2. Solve for the balanced growth path of output per capita in both models, showing all working. 3. Does the inclusion of human capital increase the rate of growth compared to the standard model? What is the impact on output in the short and long run? 1. Consider the n-times repeated game with the following stage game b 3,3 0,0 0,0 0,0 2,2 1,0 0,0 0,1 0,0 (a) Find a lower bound a for the average payoff of each player in all pure strategy Nash equilibria. Prove indeed that the payoff of a player is at least an in every pure-strategy Nash equilibrium. (Your grade will be 107.) (b) Construct a pure-strategy subgame-perfect Nash equilibrium in which the payoff of each player is at most n + 1. Verify that the strategy profile is indeed a subgame-perfect Nash equilibrium. 2. Consider the infinitely repeated game with discount factor o E (0, 1) and the stage game (1,0,0) (0,2,2) (5,0,0) (5,0,0) (2,1,1) For each of the strategy profile below, find the range of o under which the strategy profile is a subgame-perfect Nash equilibrium. (a) The play is (I, L. R) if (I, L, R) has been played at all previous days, and (X, L, [) is played otherwise. (b) On "Sundays", i.e., at t c {0, 7, 14,...}, (X, L, L) is played; on other days, (1, L, R) is played if (1, L. R) has been played at all previous such days and (X, L, L) is played otherwise. (c) On "Sundays", (X, L, L) is played; on other days, (I, R, R) is played if (I, R, R) has been played at all previous such days and (X, L, L) is played otherwise.3. Consider the infinitely repeated prisoners' dilemma game with discount factor 6 = 0.999; the stage game is C D C 5,5 0,6 D 6,0 (a) Find a subgame-perfect Nash equilibrium in pure strategies under which the average payoff of each player is in between 1.1 and 1.2. Verify that your strategy profile is indeed a subgame-perfect Nash equilibrium. (b) Find a subgame perfect Nash equilibrium in pure strategies under which the average payoff of player 1 is at least 5.7. Verify that your strategy profile is indeed a subgame- perfect Nash equilibrium. (c) Can you find a subgame-perfect Nash equilibrium under which the average payoff of player 1 is more than 5.8? 4. [Recall Problem 4 in Homework 2.] Consider the repeated game with the following stage game. A unit mass of kids are uniformly located on a street, denoted by the [0, 1] interval. There are two ice cream parlors, namely 1 and 2, located at 0 and 1, respectively. Each ice cream parlor i sets a price p. 0.' A kid located in w is to pay cost clw - yl to go to a store located at y, where ce (0, p/3). Given the prices pi and p2, each kid buys one unit of ice cream from the store with the lowest total cost, which is the sum of the price and the cost to go to the store. (If the total cost is the same, she flips a coin to choose the store to buy.) (a) Assume that the above game is repeated 100 times, and find the subgame-perfect Nash equilibria. (b) Assume that the above game is repeated infinitely many times and the discount rate is 6 E (1/3, 1). For each of the following strategy profile, find the highest p" under which the strategy profile is a subgame-perfect Nash equilibrium. (Here, p* may be a function of 6. You need to choose both p* and p to make the strategy profile a subgame-perfect Nash equilibrium.) 1. At the beginning each parlor i chooses pi = p* and continues to do so until some player sets a different price; each i selects price p; = p thereafter. 2. There are two states: Collusion and War. The game starts at the state Collusion. In Collusion state, each player i chooses p; = p*, and in War state each player i chooses pi = p. If both players set the price prescribed for the state, then the state in the next round is Collusion; the state in the next round is War otherwise. 3. Bonus: In part (ii), assume that p 2 0 but war can last multiple periods. State such a strategy profile formally and answer the above question for such a strategy profile











