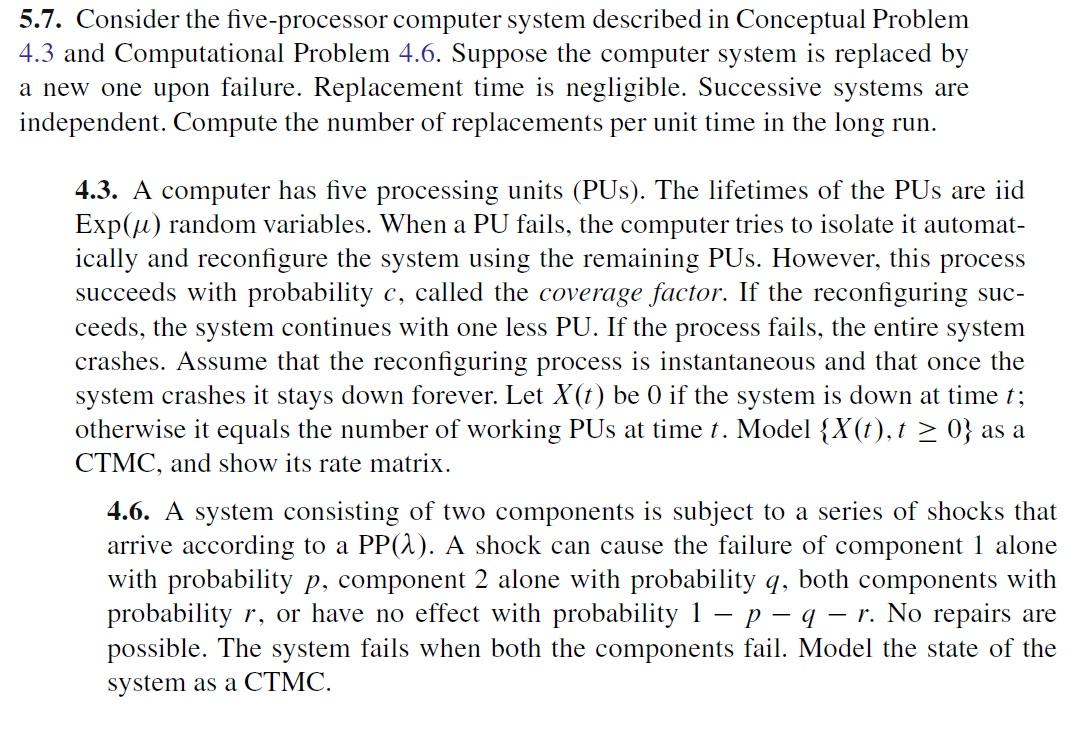
Kulkarni page 181, 5.7

5.7. Consider the five-processor computer system described in Conceptual Problem 4.3 and Computational Problem 4.6. Suppose the computer system is replaced by a new one upon failure. Replacement time is negligible. Successive systems are independent. Compute the number of replacements per unit time in the long run. 4.3. A computer has five processing units (PUs). The lifetimes of the PUs are iid Exp(u) random variables. When a PU fails, the computer tries to isolate it automat- ically and reconfigure the system using the remaining PUs. However, this process succeeds with probability c, called the coverage factor. If the reconfiguring suc- ceeds, the system continues with one less PU. If the process fails, the entire system crashes. Assume that the reconfiguring process is instantaneous and that once the system crashes it stays down forever. Let X(t) be 0 if the system is down at time t; otherwise it equals the number of working PUs at time t. Model {X(t), t > 0} as a CTMC, and show its rate matrix. 4.6. A system consisting of two components is subject to a series of shocks that arrive according to a PP(a). A shock can cause the failure of component 1 alone with probability p, component 2 alone with probability q, both components with probability r, or have no effect with probability 1 - p - q - r. No repairs are possible. The system fails when both the components fail. Model the state of the system as a CTMC. 5.7. Consider the five-processor computer system described in Conceptual Problem 4.3 and Computational Problem 4.6. Suppose the computer system is replaced by a new one upon failure. Replacement time is negligible. Successive systems are independent. Compute the number of replacements per unit time in the long run. 4.3. A computer has five processing units (PUs). The lifetimes of the PUs are iid Exp(u) random variables. When a PU fails, the computer tries to isolate it automat- ically and reconfigure the system using the remaining PUs. However, this process succeeds with probability c, called the coverage factor. If the reconfiguring suc- ceeds, the system continues with one less PU. If the process fails, the entire system crashes. Assume that the reconfiguring process is instantaneous and that once the system crashes it stays down forever. Let X(t) be 0 if the system is down at time t; otherwise it equals the number of working PUs at time t. Model {X(t), t > 0} as a CTMC, and show its rate matrix. 4.6. A system consisting of two components is subject to a series of shocks that arrive according to a PP(a). A shock can cause the failure of component 1 alone with probability p, component 2 alone with probability q, both components with probability r, or have no effect with probability 1 - p - q - r. No repairs are possible. The system fails when both the components fail. Model the state of the system as a CTMC