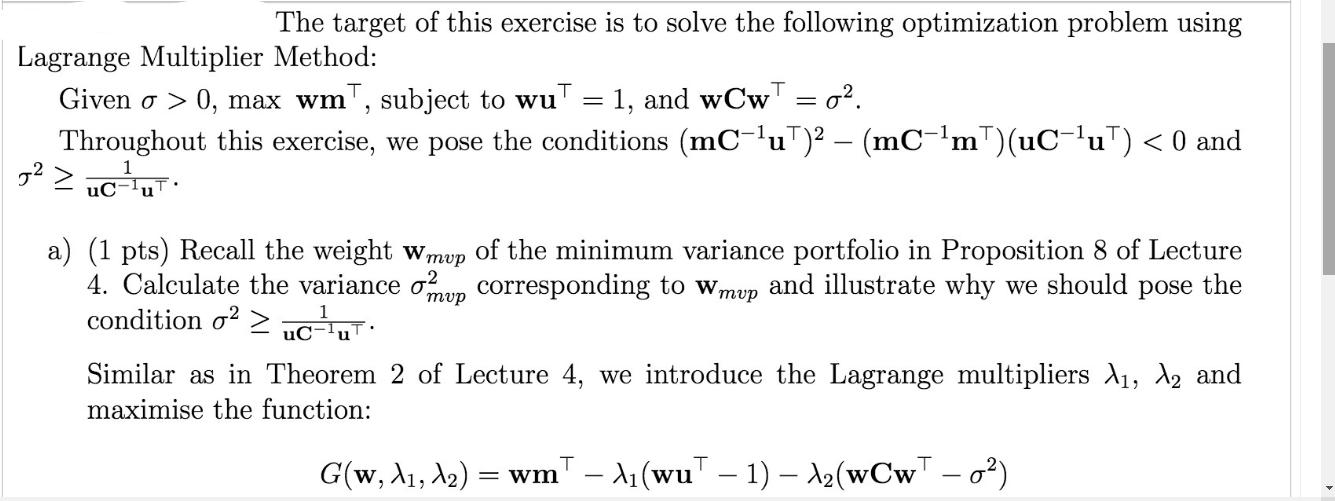
Lagrange Multiplier Method: Given o > 0, max wm, subject to wu = 1, and wCwT = . Throughout this exercise, we pose the conditions (mC-u) (mCm)(uCu) < 0 and 1 J> The target of this exercise is to solve the following optimization problem using uC a) (1 pts) Recall the weight Wmvp of the minimum variance portfolio in Proposition 8 of Lecture 4. Calculate the variance op corresponding to Wmvp and illustrate why we should pose the condition o2 uc-ut. mvp Similar as in Theorem 2 of Lecture 4, we introduce the Lagrange multipliers , 2 and maximise the function: T G(w, A, A) = wm - A (wu - 1) A (wCw - 0)