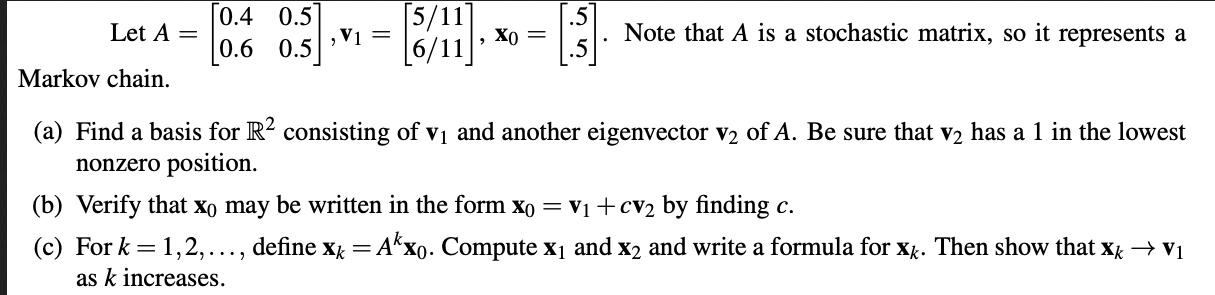
Let A = 0.4 0.5 0.6 0.5 , V = 5/11 6/11 Note that A is a stochastic matrix, so it represents a Markov chain. (a) Find a basis for R2 consisting of v and another eigenvector v of A. Be sure that v has a 1 in the lowest nonzero position. (b) Verify that xo may be written in the form Xo = V +cv by finding c. (c) For k= 1,2, ..., define xk = Akxo. Compute X and x2 and write a formula for Xk. Then show that XK V as k increases.