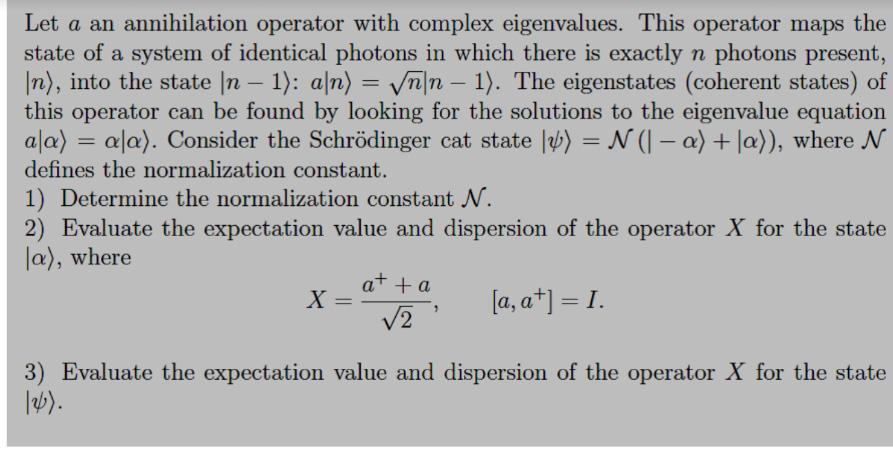
Let a an annihilation operator with complex eigenvalues. This operator maps the state of a system of identical photons in which there is exactly n photons present, |n), into the state |n 1): a|n) this operator can be found by looking for the solutions to the eigenvalue equation ala) = ala). Consider the Schrdinger cat state |) = N (| a) + la)), where N defines the normalization constant. = Vn]n 1). The eigenstates (coherent states) of %3D 1) Determine the normalization constant N. 2) Evaluate the expectation value and dispersion of the operator X for the state Ja), where at + a [a, a+] = I. V2 3) Evaluate the expectation value and dispersion of the operator X for the state |4).