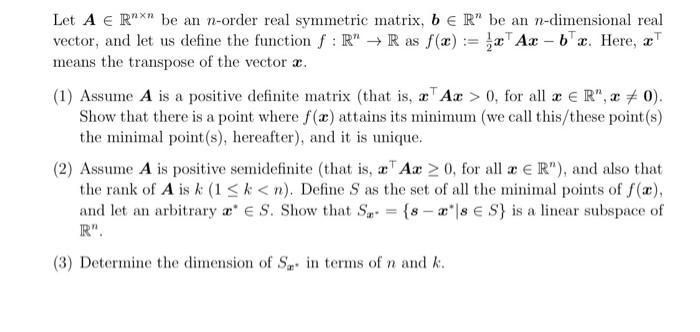
Let AR be an n-order real symmetric matrix, b = R" be an n-dimensional real vector, and let us define the function f : R" R as f(x) = x Axbx. Here, x means the transpose of the vector x. (1) Assume A is a positive definite matrix (that is, x Ax > 0, for all x R", x0). Show that there is a point where f(x) attains its minimum (we call this/these point(s) the minimal point(s), hereafter), and it is unique. (2) Assume A is positive semidefinite (that is, a Ax 0, for all a R"), and also that the rank of A is k (1 < k