Question: Let B = {sin 5x, cos 5x, x sin 5x, x cos 5x} be a basis of the vector space V = span(B). 1)

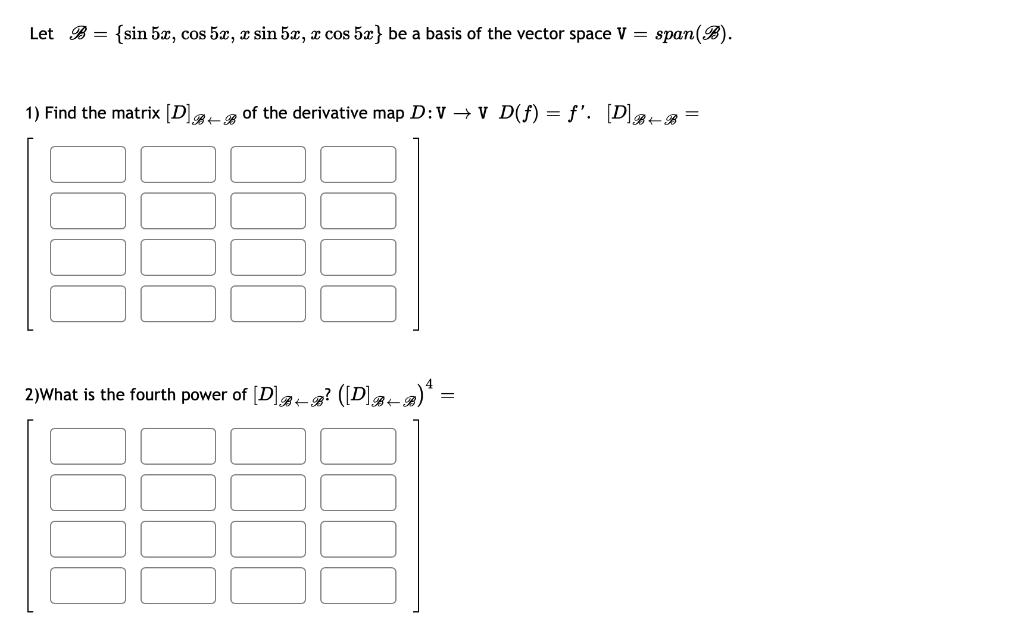
Let B = {sin 5x, cos 5x, x sin 5x, x cos 5x} be a basis of the vector space V = span(B). 1)

Let B = {sin 5x, cos 5x, x sin 5x, x cos 5x} be a basis of the vector space V = span(B). 1) Find the matrix [D]a 2 of the derivative map D:V V D(f) = f'. [D]a. 2)What is the fourth power of [D]g? ([D]-R)* = 3) Use the matrix in the previous part to find the fourth derivative of f(x) f(4) (x) = a sin 5x + Bcos 5 + y sin 5x + dx cos 5x. = 10 sin 5x + 8 cos 5x + 5x sin 5x + 3x cos 5x in the form a = B = -1 4) What is the inverse of [D]a? ([D]g+B) 5) Use the matrix in the previous part to find the coefficients p, r,s, and t in the integral (6 sin 5x + 9 cos 5x + 3x sin 5x + 8x cos 5a)dx = = p sin 5x + r cos 5x + sx sin 5x + tx cos 5x + C. p = r = s = t =

Step by Step Solution

★★★★★

3.49 Rating (152 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

Bsinsa ussa asinsa 210352 2 is the basis of the vector ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Find the dimension of the vector space V and give a basis for V. V = {A in M 22 : AB = BA}, Where B =

Let V be the subspace of the vector space of all real-valued continuous functions that has basis S = {et, e-t}. Show that V and R2 are isomorphic.

Find the dimension of the vector space V and give a basis for V. V = {A in M 22 : A is skew-symmetric}

Section 8.5 Reading Assignment: Integration of Rational Functions by Partial Fractions Answer Only Exercise 1, 2, and 3 by using a screenshot provided Calculus Pearson textbook. Make sure you read...

ay =-0. 5 - 0 . 5 = 3 x (12 ) da 0 . 5 3 x 12x 12 2-1. 6 x 10 #t/ min 4. Use the information gained from the first and second derivative test to graph the function f(x) = r + 2 cos x on [-27, 27]. Be...

Name: 20922CB8 V1 - Page 1 1) What is the quotient when x' - 2x2 - 9 is divided 6) Find the given quotient using Long Division of by x - 3? Polynomials [Show all work. ]: (4x3 - 5x2 - 16x + 20) + (4x...

Select the correct description of right-hand and left-hand behavior of the graph of the polynomial function. (x) = 4x2 - 5x + 4 Falls to the left, rises to the right. Falls to the left, falls to the...

Repeat Exercise 45 for the functions f(t) = 3 sin t - 4 sin 3 t, g(t) = 1 - 8 sin 2 t + 8 sin 4 t, h(t) = 5 sin t - 20 sin 3 t + 16 sin 5 t, in the vector space Span {I, sin t, sin 2 t, ..., sin 5...

Let R be the flow rate of a gas through a cylindrical pipe for a fixed drop in pressure between the two ends of the pipe For the following recall that you do not need to simplify or expand any of...

For the most recent year, Romero Company reported average operating assets of $200,000 and sales of $500,000. Calculate the margin Romero Company needed to earn in the most recent year in order to...

What is your contribution to the economy in terms of taxation? Explain. Do you think the government is doing its best to collect taxes in order to support the health and safety of the people? Explain...

find the limit 7 u ^ 2 / u - 2

Over The Edge (OTE) is a good candidate for applying the capitalized cash flow approach of business valuation. In your valuation spreadsheets, be sure to make notes explaining each of your...

As the senior accountant at Active Quality Sportswear (AQS), which manufactures sport attire for men, women, youth, and children, you are often asked to prepare various financial analysis necessary...

On October 1, 2017, Darcy Zaylo organized a computer service I need this assignment.do you a solution for this assignment? Darcy Systems On October 1, 2017, Darcy Zaylo organized a computer service...

On October 1, 2017, Darcy Zaylo organized a computer service company called Darcy Systems. Darcy is organized as a sole proprietorship and will provide consulting services, computer system...

= Consider the following discrete time two-period market model. The savings account is given by t 5 55 where and are 51 t for t= 0, 1, 2. The stock price is given by So = 1 and S = and S =...

solve Question 9 (1 point) Damage to DNA: J a) is only caused by external contaminants. O b) can be repaired in the cell. c) cannot be repaired. d) is only caused by synthetic pesticides. O e) all of...

Let B = {sin 4x, cos 4x, x sin 4x, x cos 4x} be a basis of the vector space V = span(B). 1) Find the matrix [D]+B of the derivative map D:V V D(f) = f'. [D]g+ 4 2)What is the fourth power of [D]g g?...

Where are the extrema for the function f ( a sin (c) 2-cos (I) for x in [-7, 7]? O A. relative minimum: (0, 0) , relative maximum: (", ?) O B. relative minimum: (- , relative maximum: 3 O C. relative...

for part 5 chose one a or b 1. The following matrices are related. A' is row-equivalent to A, and A" is the transpose of A. Use this information to complete the following: N 2 1 -6 00 Co its co 3 A =...

I need answers for questions 7-9 You notice that the flow of the water alternates between two flows that we'll call flow 1 and flow 2. The x and y coordinates are as shown above. The velocity v of...

1. Find the vector equation of a line in IR that passes through the points P(3, 6, 0) and Q(-2, 3, 1). O l(t) = (-5t, -3t, t) O l(t) = (-2 + 3t, 3 + 6t, 1) O e(t) = (-2+5t, 3 +3t, 1 -t) O e(t) =...

please help me with this asap do it carefully and clearly plz. # Import library import numpy as #(2 points) # Function register_Arun_3D # Input: Two 3x matrices of points to register: # fixed dataset...