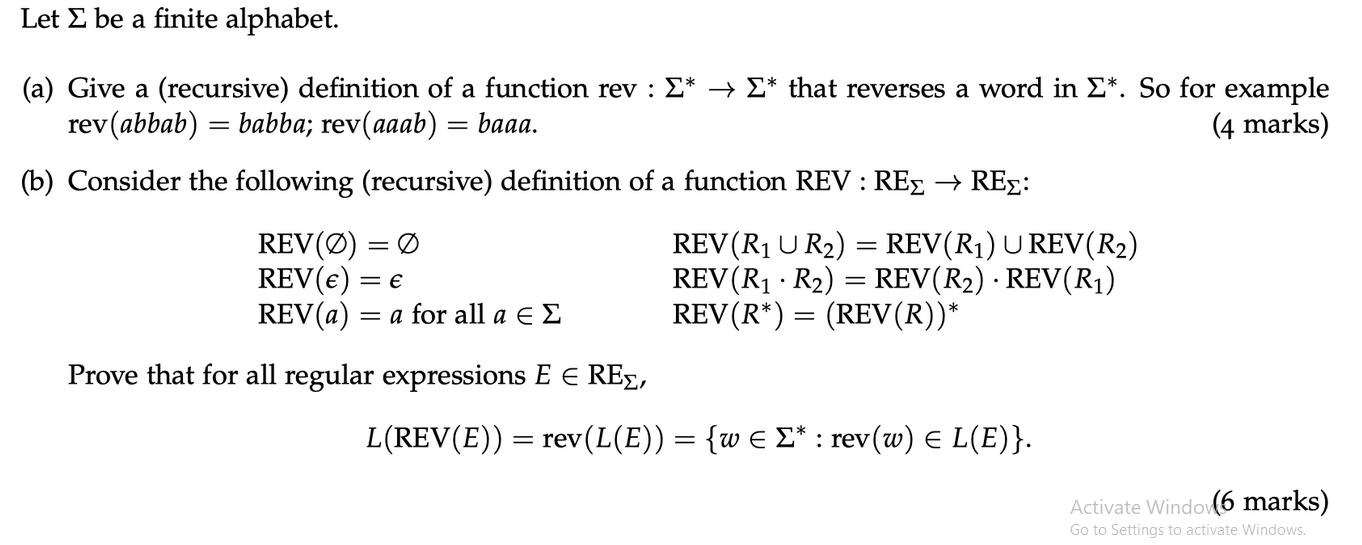
Let be a finite alphabet. (a) Give a (recursive) definition of a function rev : * * that reverses a word in *. So for example rev (abbab) = babba; rev(aaab) = baaa. (b) Consider the following (recursive) definition of a function REV: RE RE: (4 marks) REV(0) = 0 REV() = . = REV (R UR2) = REV (R) U REV(R2) REV (R1 R2) REV (R2) REV(R1) REV(a) = a for all a Prove that for all regular expressions E = RE, REV (R*) = (REV (R))* L(REV(E)) = rev(L(E)) = {w * : rev(w) L(E)}. Activate Window (6 marks) Go to Settings to activate Windows.