Question: Let C be a binary linear code of length n. As usual, we write w(x) for the weight of codeword x, d(x, y) =
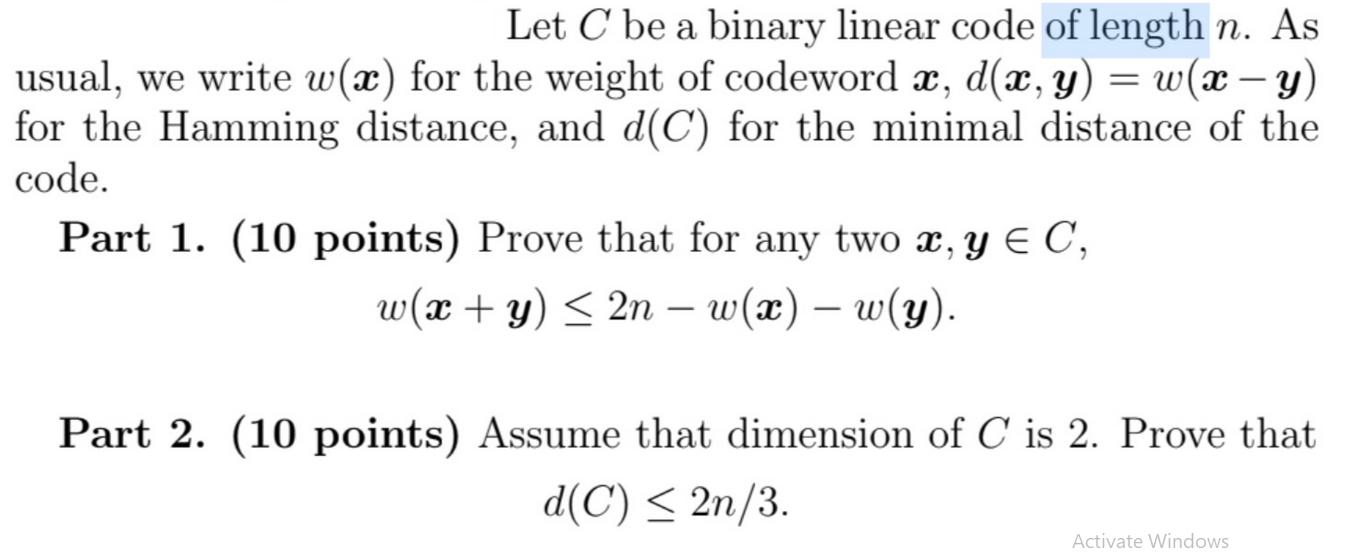
Let C be a binary linear code of length n. As usual, we write w(x) for the weight of codeword x, d(x, y) = w(x - y) for the Hamming distance, and d(C) for the minimal distance of the code. Part 1. (10 points) Prove that for any two x, y E C, w(x+y) 2n - w(x) w(y). - Part 2. (10 points) Assume that dimension of C is 2. Prove that d(C) 2n/3. Activate Windows
Step by Step Solution
3.47 Rating (160 Votes )
There are 3 Steps involved in it
Part 1 10 points Prove that for any two x y C wx y 2n wx wy We want to prove that for any two codewords x y C w x y 2 n w x w y Lets denote xi and yi as the ith components of x and y respectively for ... View full answer
Get step-by-step solutions from verified subject matter experts
Study Help