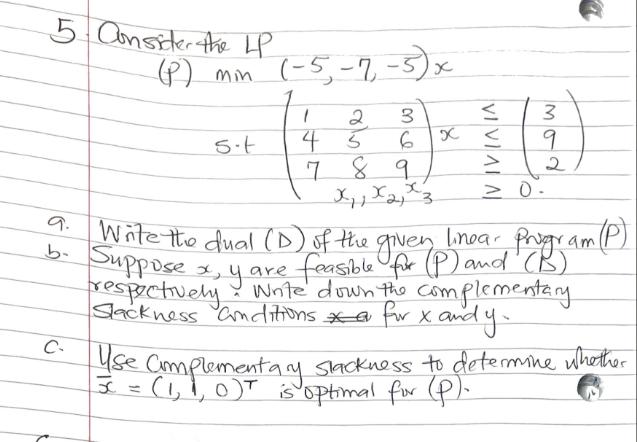
Let c, d E TR". Consider the LP. (P) max St. dx = 100 Describe the conditions on c under which (P) would have an optimal solution, and prove your claim- D -nxn Where A = - A 3. Suppose bt R", and AtR (these are usually called Skew - Symmetric matices). Consider the LP- (P) max bTx St. -b x = 0 Construct the dual (D) of (P) (b) Prove that every feasible solution in (P) has non- Positive (ie, O or lower Objective value. 4. Let A be an mxn matrix, bt Rm and CER". Consider The LPs B (P2 maxcx Subject to Ax b, x 0. max cTx Subject to Ax = b, x0. Suppose we know that (P) has an optimal solution, but (P2) is infeasible. Show that the dual of (P2) mal solution must be unbounded- 5. Consider the LP 9. (P) min (-5-7-5)x 5-t ' 45 2 3 3 6 X 9 > 2 789 x1, x2, x3 Write the dual (D) of the given linear Program (P) 5. Suppose x, y are feasible for (P) and (B) respectively. Write down the complementary Slackness Conditions *a for x and y. Use Complementary slackness to determine whether sc = (1,1,0)T is optimal for (p).