Question: Let Enc() be an public-key encryption scheme that is additively homo- morphic. (a) Let Zn be the space of messages of Enc(). Let p(x)

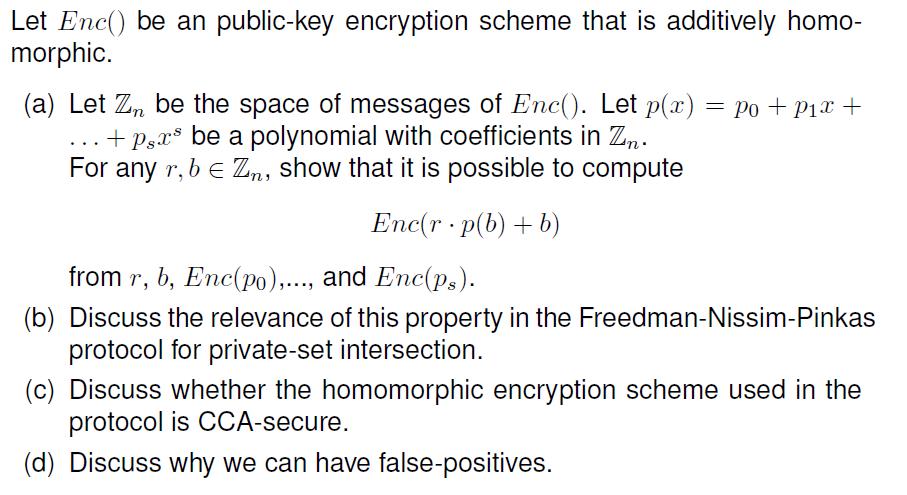
Let Enc() be an public-key encryption scheme that is additively homo- morphic.

Let Enc() be an public-key encryption scheme that is additively homo- morphic. (a) Let Zn be the space of messages of Enc(). Let p(x) = po + Px + +psas be a polynomial with coefficients in Zn. For any r, b Zn, show that it is possible to compute Enc(r p(b) + b) ... from r, b, Enc(po),..., and Enc(ps). (b) Discuss the relevance of this property in the Freedman-Nissim-Pinkas protocol for private-set intersection. (c) Discuss whether the homomorphic encryption scheme used in the protocol is CCA-secure. (d) Discuss why we can have false-positives.

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Programming Questions!

Analyze Rivalry Among Existing Competitors Describe the rivalry among existing competitors in the new industry you've identified (The new industry that would be a good fit for our company is Electric...

can someone solve this Modern workstations typically have memory systems that incorporate two or three levels of caching. Explain why they are designed like this. [4 marks] In order to investigate...

Let A, B be sets. Define: (a) the Cartesian product (A B) (b) the set of relations R between A and B (c) the identity relation A on the set A [3 marks] Suppose S, T are relations between A and B, and...

If an encryption scheme has CPA security then it also has CPAS security. 2 pts True False Question 7 Let Enc be a CPA-secure encryption scheme. Then running Enc(k,m) twice (with the same k, m ) will...

URGENT SOLUTION REQUIRED COURSE: Information security aur topic cryptography 1. Public-Key Encryption (25 points) Let (Gen, Enc, Dec) be a public-key encryption that is...

You are given a (CPA-secure) Public-key encryption scheme E=(GenE, Enc, Dec), a (securet) digital signature scheme S=(Gens, Sig, rfys) and a (securet) private-key authentication scheme A=(Gen_A,...

Ffffff Slide 1 2 DEFINITION 1 2 . 1 A public - key encryption scheme is a triple of proba - bilistic polynomial - time algorithms ( Gen , Enc, Dec ) such that: The key - generation algorithm Gen...

CONSTRUCTION 1 2 . 3 6 Let GenRSA be as in the previous sections, and l , k be as described in the text. Let G : { 0 , 1 } k - > { 0 , 1 } e + k and H : { 0 , 1 7 \ deg + k - > { 0 , 1 } k be...

CONSTRUCTION 1 2 . 1 6 Let 9 be as in the text. Define a public - key encryption scheme as follows: Gen: on input 1 " run G ( 1 " ) to obtain ( G , q , g ) . Then choose a uniform a Zq and compute h...

Cryptography Question from Katz and Lindell Book Introduction to Modern Cryptography For each of the following encryption schemes, state whether he scheme achieves perfect secrecy. Justify your...

Under which type of inventory system is an inventory subsidiary ledger maintained?

Are the following statements true or false? Explain your answers. a. The elasticity of demand is the same as the slope of the demand curve. b. The cross-price elasticity will always be positive. c....

This describes investment opportunity in detail to appeal to buyers on the buyside what is a prospectus? what is a stock pitch? what is a company sales pitch?

The following is a payroll sheet for Bayview Golf Corporation for the month of September 2011. The employment insurance rate is 1.73% and the maximum annual amount per employee is $747.36. The...

Sally was an all-state soccer player during her junior and senior years in high school. She accepted an athletic scholarship from State University. The scholarship provided the following: Tuition and...

At the beginning of the year, office supplies of $ 1,100 were on hand. During the year, Dash Air Conditioning Service paid $ 5,000 for more office supplies. At the end of the year, Dash has $ 700 of...

Two point charges are located on the y-axis as follows: charge q1 = -1.50 nC at y = -0.600m, and charge q2 = +3.20 nC at the origin (y = 0). What is the total force (magnitude and direction) exerted...

The following data at July 31, 2014, are given for EBM. a. Depreciation, $ 500. b. Prepaid rent expires, $ 600. c. Interest expense accrued $ 300. d. Employee salaries owed for Monday through...

The return of goods to the supplier will affect the purchase account. Question 27Answer True False

a) Prove that the improper integral «£° e-x2 dx converges to a finite real number. b) Prove that if f is the value of the integral in part a), then c) Show that d) Let Qk represent...

Suppose that E and V are subsets of R with E bounded, V open, and V. Prove that there is a C function F: E R such that F(x) > 0 for x E and f(x) = 0 for V.

Suppose that E R" is connected and that E A . Prove that A is connected.

It was hypothesized that road construction workers do not engage in productive work 20 minutes on the average out of every hour. Some claimed the nonproductive time is greater than 20 minutes. An...

For your e-mail, you use a filter to block spam from your inbox. The number of items blocked by day of week is recorded and Minitab is used to perform the analysis that follows. Here are the results:...

The City of Maumee comprises four districts. Chief of Police Andy North wants to determine whether there is a difference in the mean number of crimes committed among the four districts. He examined...