Solve the following questions

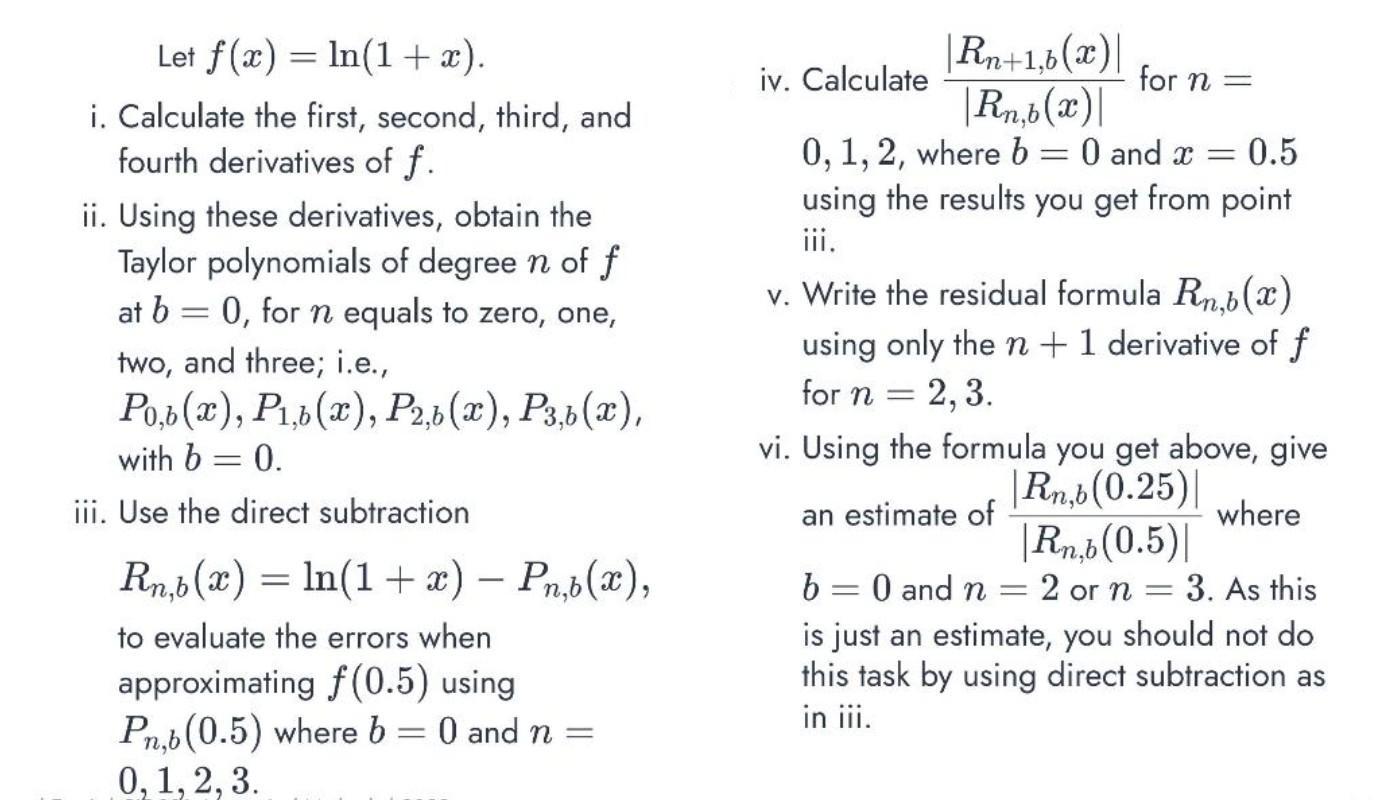
Let f(x) = ln(1 + x). i. Calculate the first, second, third, and fourth derivatives of f. ii. Using these derivatives, obtain the Taylor polynomials of degree n of f at b = 0, for n equals to zero, one, two, and three; i.e., Po,b(x), P,b(x), P2,6(x), P3,6(x), with b = 0. iii. Use the direct subtraction Rnb (x) = ln(1 + x) = ln(1+x) - - Pn,b(x), to evaluate the errors when approximating f(0.5) using Pn,b (0.5) where b 0 and n = 0, 1, 2, 3. = iv. Calculate | Rn+1,b(x) |Rn.b(x)| for n = 0, 1, 2, where b = 0 and x = 0.5 using the results you get from point iii. v. Write the residual formula Rn,b(x) using only the n + 1 derivative of f for n = 2, 3. vi. Using the formula you get above, give Rnb (0.25) where |Rn,b(0.5)| an estimate of = 2 or n = 3. As this = b = 0 and n is just an estimate, you should not do this task by using direct subtraction as in iii.