Question
Let G = (V, E) be an undirected graph on the nodes V = {1, . . . , n}. Suppose the edges of the
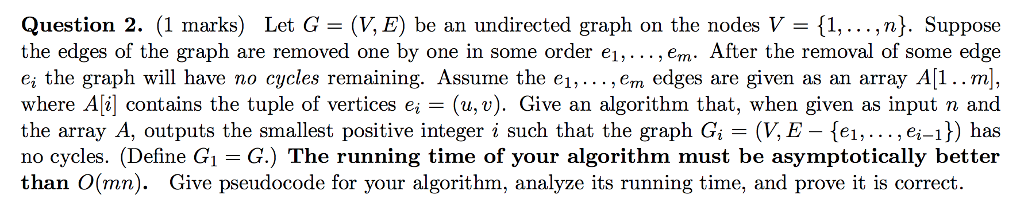 Let G = (V, E) be an undirected graph on the nodes V = {1, . . . , n}. Suppose the edges of the graph are removed one by one in some order e1, . . . , em. After the removal of some edge ei the graph will have no cycles remaining. Assume the e1, . . . , em edges are given as an array A[1 . . m], where A[i] contains the tuple of vertices ei = (u, v). Give an algorithm that, when given as input n and the array A, outputs the smallest positive integer i such that the graph Gi = (V, E {e1, . . . , ei1}) has no cycles. (Define G1 = G.) The running time of your algorithm must be asymptotically better than O(mn). Give pseudocode for your algorithm, analyze its running time, and prove it is correct
Let G = (V, E) be an undirected graph on the nodes V = {1, . . . , n}. Suppose the edges of the graph are removed one by one in some order e1, . . . , em. After the removal of some edge ei the graph will have no cycles remaining. Assume the e1, . . . , em edges are given as an array A[1 . . m], where A[i] contains the tuple of vertices ei = (u, v). Give an algorithm that, when given as input n and the array A, outputs the smallest positive integer i such that the graph Gi = (V, E {e1, . . . , ei1}) has no cycles. (Define G1 = G.) The running time of your algorithm must be asymptotically better than O(mn). Give pseudocode for your algorithm, analyze its running time, and prove it is correct
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


