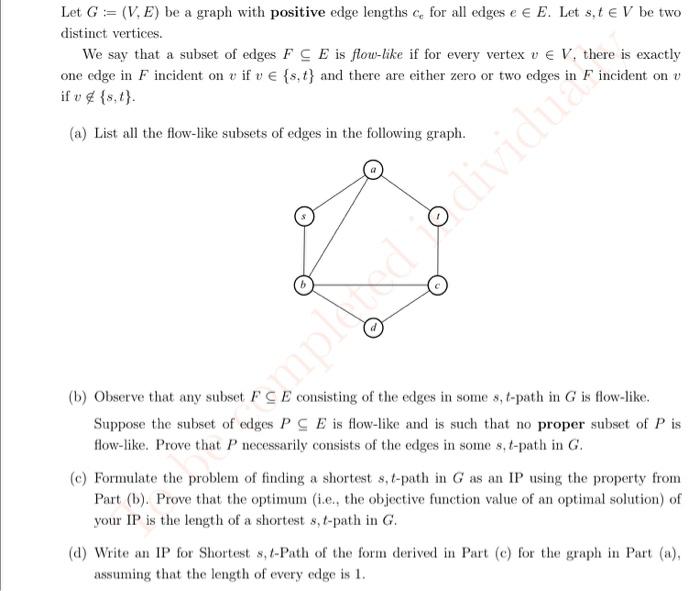
Let G = (V,E) be a graph with positive edge lengths ce for all edges e E. Let sit V be two distinct vertices. We say that a subset of edges F C E is flow-like if for every vertex v V, there is exactly one edge in F incident on vif ve {s, t} and there are either zero or two edges in F incident on v if v{s,t). (a) List all the flow-like subsets of edges in the following graph. mpled odividu (b) Observe that any subset FCE consisting of the edges in some s, t-path in G is flow-like. Suppose the subset of edges PCE is flow-like and is such that no proper subset of P is flow-like. Prove that P necessarily consists of the edges in some s, t-path in G. (c) Formulate the problem of finding a shortest s, t-path in G as an IP using the property from Part (b). Prove that the optimum (i.e., the objective function value of an optimal solution) of your IP is the length of a shortest s, t-path in G. (d) Write an IP for Shortest s, t-Path of the form derived in Part (c) for the graph in Part (a), assuming that the length of every edge is 1.