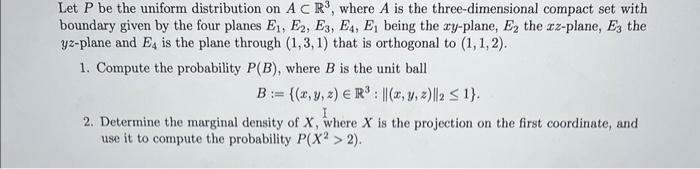
Let P be the uniform distribution on A CR, where A is the three-dimensional compact set with boundary given by the four planes E1, E2, E3, E4, E being the zy-plane, E2 the zz-plane, E3 the yz-plane and E4 is the plane through (1,3,1) that is orthogonal to (1,1,2). 1. Compute the probability P(B), where B is the unit ball B:= {(x, y, z) E R: ||(x, y, z)||2 < 1). 2. Determine the marginal density of X, where X is the projection on the first coordinate, and use it to compute the probability P(X2 > 2).