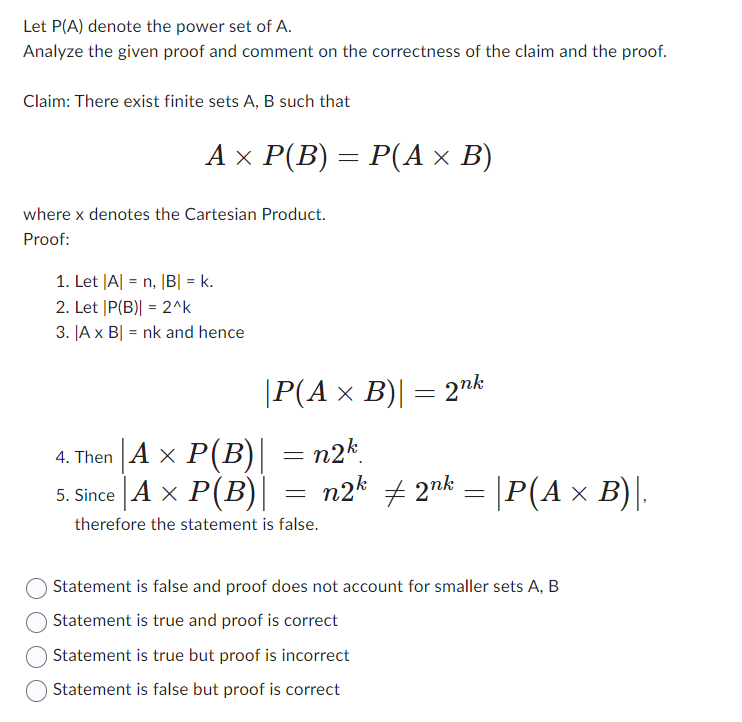
Let P(A) denote the power set of A. Analyze the given proof and comment on the correctness of the claim and the proof. Claim: There exist finite sets A, B such that A P(B) = P(A B) where x denotes the Cartesian Product. Proof: 1. Let |A| =n, |B| = k. 2. Let | P(B) = 2^k 3. A x B=nk and hence |P(A B)| = 2nk 4. Then |A P(B)| = n2k. 5. Since |A P(B)| therefore the statement is false. = n2k 2nk = |P(A B)|, Statement is false and proof does not account for smaller sets A, B Statement is true and proof is correct Statement is true but proof is incorrect Statement is false but proof is correct