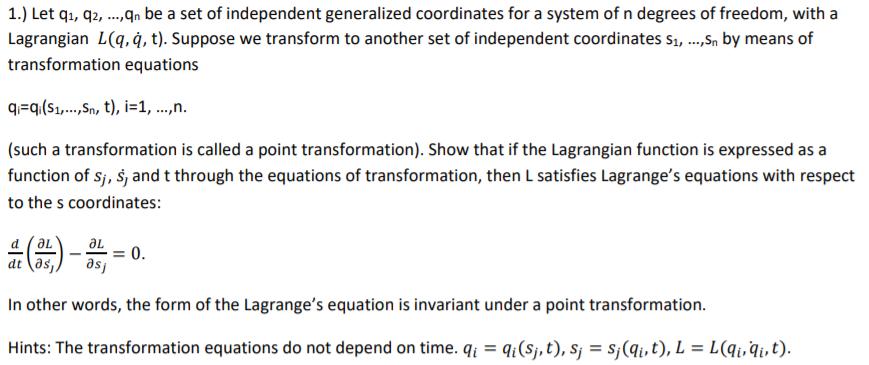
Let q, 92, ...,qn be a set of independent generalized coordinates for a system of n degrees of freedom, with a Lagrangian L(q, q, t). Suppose we transform to another set of independent coordinates S, ...,Sn by means of transformation equations qiqi(S,...,Sn, t), i=1,...,n. (such a transformation is called a point transformation). Show that if the Lagrangian function is expressed as a function of sj, s, and t through the equations of transformation, then L satisfies Lagrange's equations with respect to the s coordinates: L asj In other words, the form of the Lagrange's equation is invariant under a point transformation. Hints: The transformation equations do not depend on time. qi = ai (Sj, t), SjSj (qi, t), L = L(qi, qi, t). L dtas, = 0.