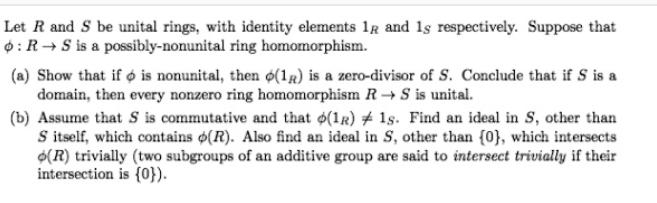
Let R and S be unital rings, with identity elements 1 and 1s respectively. Suppose that : R S is a possibly-nonunital ring homomorphism. (a) Show that if is nonunital, then (1) is a zero-divisor of S. Conclude that if S is a domain, then every nonzero ring homomorphism RS is unital. (b) Assume that S is commutative and that (1R) 1s. Find an ideal in S, other than S itself, which contains (R). Also find an ideal in S, other than (0), which intersects (R) trivially (two subgroups of an additive group are said to intersect trivially if their intersection is {0}).