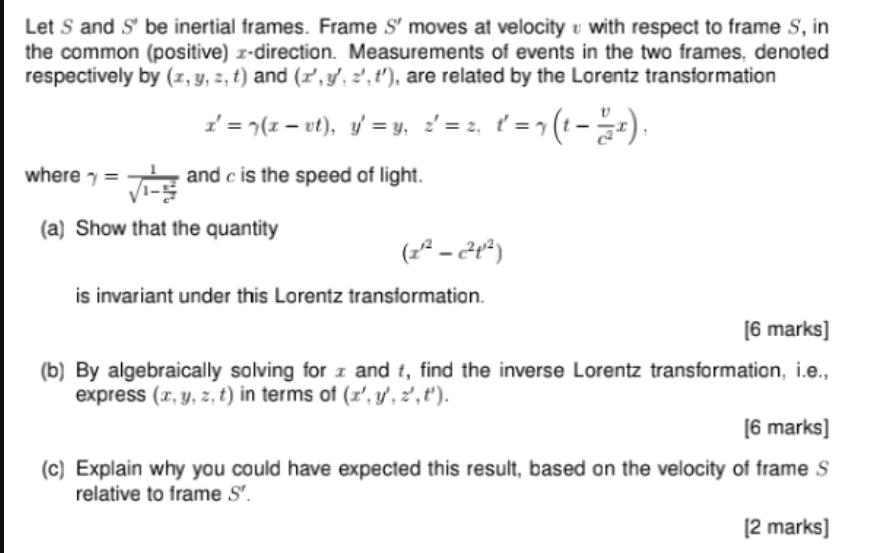
Let S and S be inertial frames. Frame S' moves at velocity with respect to frame S, in the common (positive) z-direction. Measurements of events in the two frames, denoted respectively by (z,y, z, t) and (x, y, z', t'), are related by the Lorentz transformation x' = 7(x vt), y' =y, 2' = 2, t' = q (1-2x), and is the speed of light. where = (a) Show that the quantity (z-c4) is invariant under this Lorentz transformation. [6 marks] (b) By algebraically solving for x and t, find the inverse Lorentz transformation, i.e., express (z, y, z, t) in terms of (z', y, z, t'). [6 marks] (c) Explain why you could have expected this result, based on the velocity of frame S relative to frame S'. [2 marks]