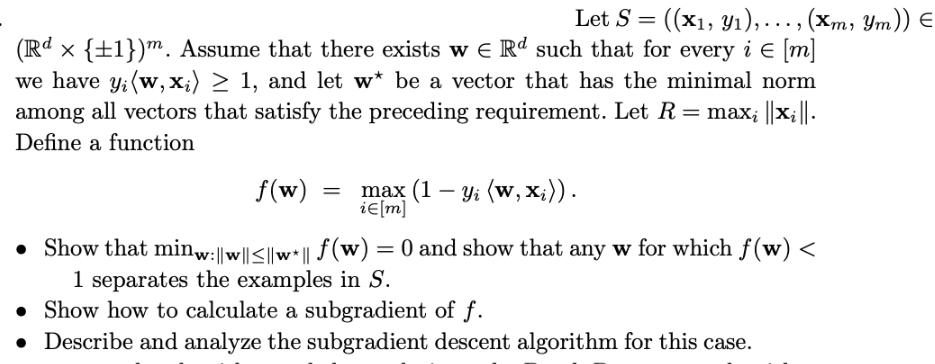
Let S ((x1,y1),..., (xm, Ym)) = (Rdx{1}). Assume that there exists w Rd such that for every i = [m] we have y (w,x) 1, and let w* be a vector that has the minimal norm among all vectors that satisfy the preceding requirement. Let R = maxi ||xi||. Define a function f(w) = max (1 yi (w, xi)). i[m] Show that minw:||w||||w* || f(w) = 0 and show that any w for which f(w) < 1 separates the examples in S. Show how to calculate a subgradient of f. Describe and analyze the subgradient descent algorithm for this case.