Let { sn } be a sequence; consider an infinite sequence of integers nj

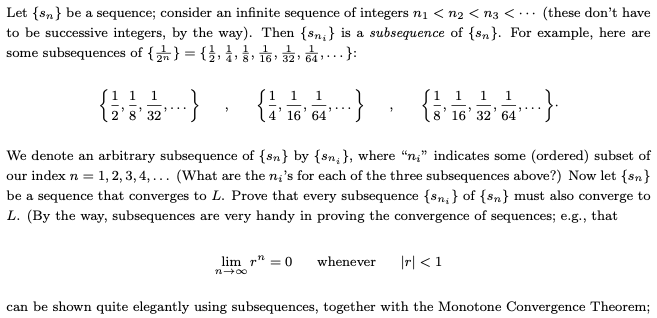
Let {sn} be a sequence; consider an infinite sequence Of integers n] < < to be successive integers, by the way). Then {sni} is a subsequence Of {sn}. some subsequences of { k} = e } : < (these don't have For example, here are {Ill 2'8'32' r 1 11 8' 16' 32' 64' We denote an arbitrary subsequence Of {sn} by } , where indicates some (ordered) subset Of our index n = I, 2, 3, 4, (What are the for each Of the three subsequences above?) Now let {so} be a sequence that converges to L. Prove that every subsequence {sn,} Of {so} must also converge to L. (By the way, subsequences are very handy in proving the convergence Of sequences; e.g., that lim r n = O whenever r I < I can be shown quite elegantly using subsequences, together with the Monotone Convergence Theorem; Let {sn} be a sequence; consider an infinite sequence Of integers n] < < to be successive integers, by the way). Then {sni} is a subsequence Of {sn}. some subsequences of { k} = e } : < (these don't have For example, here are {Ill 2'8'32' r 1 11 8' 16' 32' 64' We denote an arbitrary subsequence Of {sn} by } , where indicates some (ordered) subset Of our index n = I, 2, 3, 4, (What are the for each Of the three subsequences above?) Now let {so} be a sequence that converges to L. Prove that every subsequence {sn,} Of {so} must also converge to L. (By the way, subsequences are very handy in proving the convergence Of sequences; e.g., that lim r n = O whenever r I < I can be shown quite elegantly using subsequences, together with the Monotone Convergence Theorem;