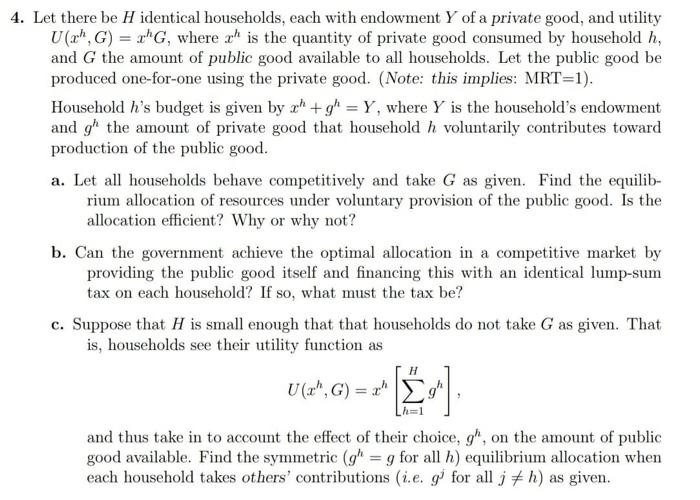
Let there be H identical households, each with endowment Y of a private good, and utility U(xh,G)=xhG, where xh is the quantity of private good consumed by household h, and G the amount of public good available to all households. Let the public good be produced one-for-one using the private good. (Note: this implies: MRT=1). Household h 's budget is given by xh+gh=Y, where Y is the household's endowment and gh the amount of private good that household h voluntarily contributes toward production of the public good. a. Let all households behave competitively and take G as given. Find the equilibrium allocation of resources under voluntary provision of the public good. Is the allocation efficient? Why or why not? b. Can the government achieve the optimal allocation in a competitive market by providing the public good itself and financing this with an identical lump-sum tax on each household? If so, what must the tax be? c. Suppose that H is small enough that that households do not take G as given. That is, households see their utility function as U(xh,G)=xh[h=1Hgh], and thus take in to account the effect of their choice, gh, on the amount of public good available. Find the symmetric (gh=g for all h) equilibrium allocation when each household takes others' contributions (i.e. gj for all j=h ) as given. Let there be H identical households, each with endowment Y of a private good, and utility U(xh,G)=xhG, where xh is the quantity of private good consumed by household h, and G the amount of public good available to all households. Let the public good be produced one-for-one using the private good. (Note: this implies: MRT=1). Household h 's budget is given by xh+gh=Y, where Y is the household's endowment and gh the amount of private good that household h voluntarily contributes toward production of the public good. a. Let all households behave competitively and take G as given. Find the equilibrium allocation of resources under voluntary provision of the public good. Is the allocation efficient? Why or why not? b. Can the government achieve the optimal allocation in a competitive market by providing the public good itself and financing this with an identical lump-sum tax on each household? If so, what must the tax be? c. Suppose that H is small enough that that households do not take G as given. That is, households see their utility function as U(xh,G)=xh[h=1Hgh], and thus take in to account the effect of their choice, gh, on the amount of public good available. Find the symmetric (gh=g for all h) equilibrium allocation when each household takes others' contributions (i.e. gj for all j=h ) as given