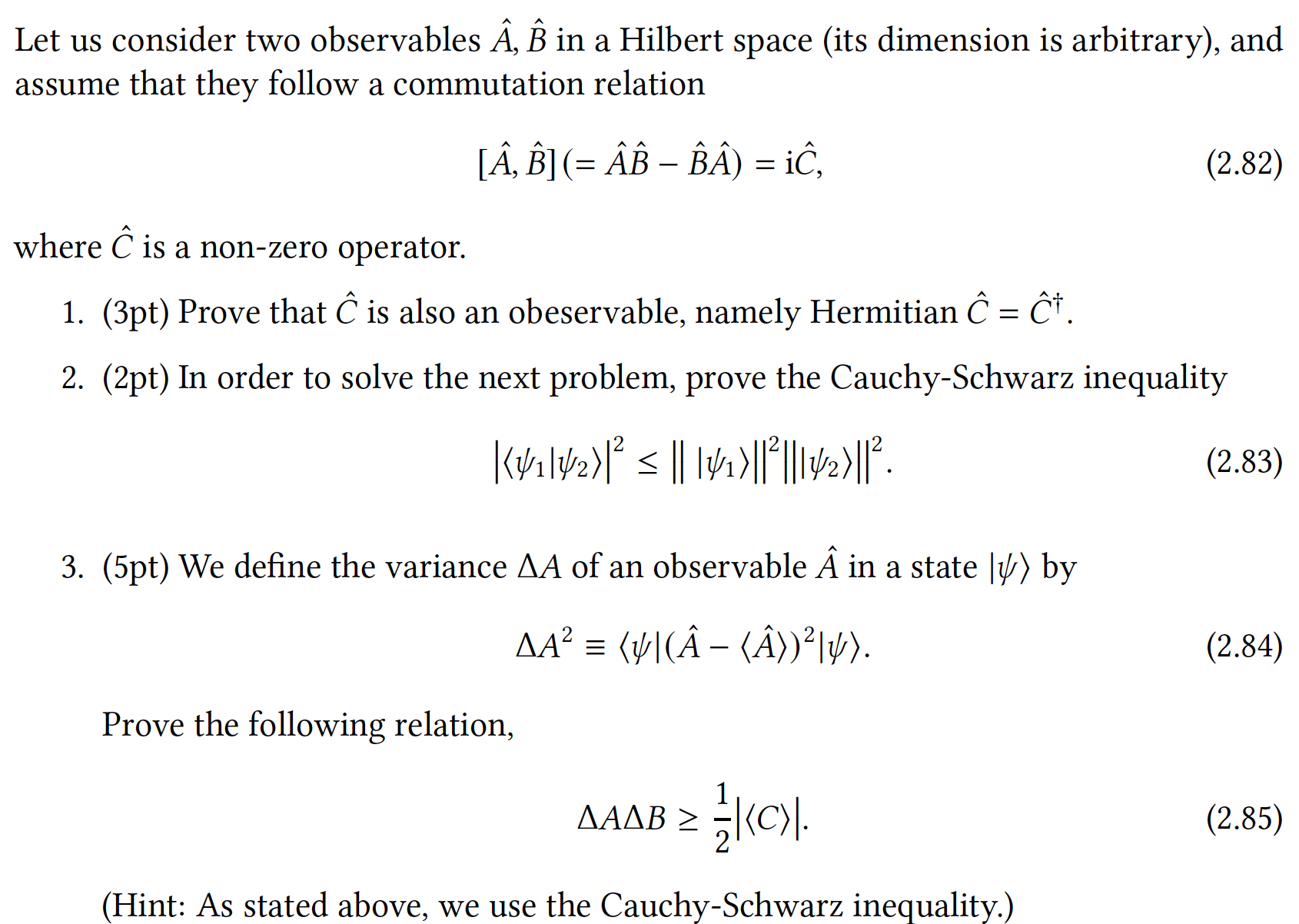
Let us consider two observables , in a Hilbert space (its dimension is arbitrary), and assume that they follow a commutation relation [, ] (= ) = i, - (2.82) where is a non-zero operator. 1. (3pt) Prove that is also an obeservable, namely Hermitian = . 2. (2pt) In order to solve the next problem, prove the Cauchy-Schwarz inequality |(4142) |||41)|||||4/2>||. 3. (5pt) We define the variance AA of an observable in a state |1/) by (2.83) AA = (|( ())|). Prove the following relation, (Hint: As stated above, we use the Cauchy-Schwarz inequality.) (2.84) (2.85)