Question
Let us define some notations: T = {to, ti, , tn} a set of n+1 distinct real numbers chosen once and for all, then define
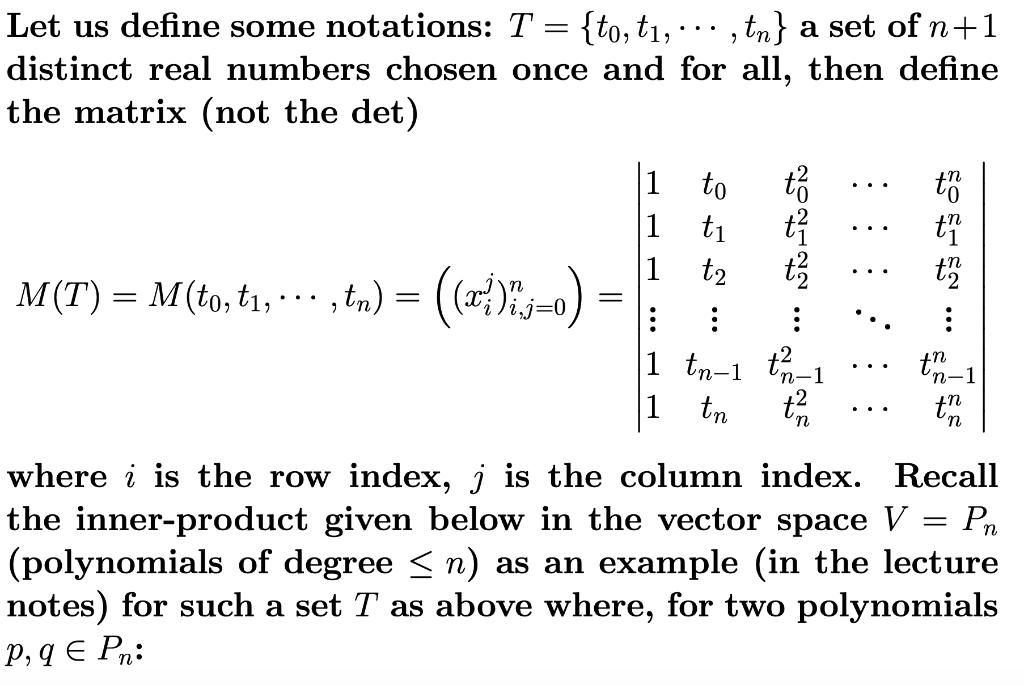

t can be expressed as = Xt AY (matrix multiplication), where X [p]s, Y = [q]s are the of Pn (show there must be some A such that...). 2) Determine A explicitly (it is related to M(T) defined above, find the exact relation to it) and verify that A is symmetric as it is supposed to be. Let us define some notations: T = {to, ti, , tn} a set of n+1 distinct real numbers chosen once and for all, then define the matrix (not the det) M(T) = M(to, ti, ,ton) = ((*)=o) = 1 to to 1 ti t 1 t2 : : : 1 tn-1 thai tn t to th th : tn_ tn in-1 n where i is the row index, j is the column index. Recall the inner-product given below in the vector space V = Pn (polynomials of degree t can be expressed as = Xt AY (matrix multiplication), where X [p]s, Y = [q]s are the of Pn (show there must be some A such that...). 2) Determine A explicitly (it is related to M(T) defined above, find the exact relation to it) and verify that A is symmetric as it is supposed to be
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


