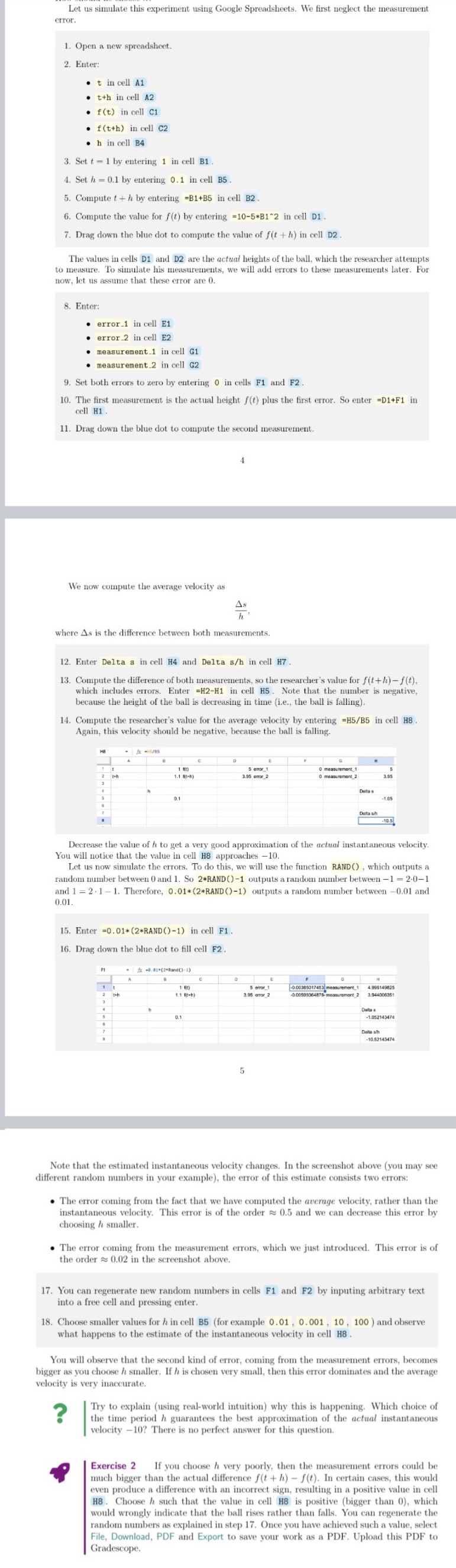
Let us simulate this experiment using Google Spreadsheets. We first neglect the measurement error. 1. Open a new spreadsheet. 2. Enter: t in cell A1 tth in cell A2 f(t) in cell c1 f(tth) in cell C2 h in cell B4 3. Set t - 1 by entering 1 in cell B1 . . Set h = 0.1 by entering 0.1 in cell B5 . 5. Compute t + h by entering =B1+85 in cell B2 6. Compute the value for f(t) by entering =10-5-B1-2 in cell D1 . 7. Drag down the blue dot to compute the value of f (t + h) in cell D2. The values in cells Di and D2 are the actual heights of the ball, which the researcher attempts to measure. To simulate his measurements, we will add errors to these measurements later. For now, let us assume that these error are 0. 8. Enter: . error-1 in cell E1 error_2 in cell E2 measurement_1 in cell G1 measurement_2 in cell G2 9. Set both errors to zero by entering 0 in cells F1 and F2 10. The first measurement is the actual height f(t) plus the first error. So enter =D1+F1 in cell H1 . 11. Drag down the blue dot to compute the second measurement. We now compute the average velocity as As where As is the difference between both measurements. 12. Enter Delta s in cell H4 and Delta s/h in cell #7. 13. Compute the difference of both measurements, so the researcher's value for f (t+h) - f(t), which includes errors. Enter =H2-H1 in cell H5 . Note that the number is negative, because the height of the ball is decreasing in time (i.e., the ball is falling). 14. Compute the researcher's value for the average velocity by entering =H5/B5 in cell H8 . Again, this velocity should be negative, because the ball is falling. 2.95 emo Decrease the value of h to get a very good approximation of the actual instantaneous velocity. You will notice that the value in cell H8 approaches -10. Let us now simulate the errors. To do this, we will use the function RANDO) , which outputs a random number between 0 and 1. So 2+RAND()-1 outputs a random number between -1 = 2.0-1 and 1 = 2. 1 - 1. Therefore, 0.01* (2*RAND()-1) outputs a random number between -0.01 and 0.01 15. Enter =0.01* (2*RAND()-1) in cell F1 . 16. Drag down the blue dot to fill cell F2. :0.00385017453/mea 3.95 error 0.60509364878 me 4.996149625 Delta s -1.052143474 -10.52143474 Note that the estimated instantaneous velocity changes. In the screenshot above (you may see different random numbers in your example), the error of this estimate consists two errors: . The error coming from the fact that we have computed the average velocity, rather than the instantaneous velocity. This error is of the order ~ 0.5 and we can decrease this error by choosing h smaller. The error coming from the measurement errors, which we just introduced. This error is of the order ~ 0.02 in the screenshot above. 17. You can regenerate new random numbers in cells F1 and F2 by inputing arbitrary text into a free cell and pressing enter. 18. Choose smaller values for h in cell B5 (for example 0.01 , 0.001 , 10 , 100 ) and observe what happens to the estimate of the instantaneous velocity in cell H8 . You will observe that the second kind of error, coming from the measurement errors, becomes bigger as you choose h smaller. If h is chosen very small, then this error dominates and the average velocity is very inaccurate. Try to explain (using real-world intuition) why this is happening. Which choice of the time period h guarantees the best approximation of the actual instantaneous velocity -10? There is no perfect answer for this question. Exercise 2 If you choose h very poorly, then the measurement errors could be much bigger than the actual difference f(t + h) - f(t). In certain cases, this would even produce a difference with an incorrect sign, resulting in a positive value in cell H8 . Choose h such that the value in cell H8 is positive (bigger than 0), which would wrongly indicate that the ball rises rather than falls. You can regenerate the random numbers as explained in step 17. Once you have achieved such a value, select File, Download, PDF and Export to save your work as a PDF. Upload this PDF to Gradescope