FREE Trial
Answered step by step
Verified Expert Solution
Link Copied!

Question

1 Approved Answer

Let X be a metric space and C = {FCX | ECF and F is closed}. If E = EUE'. Prove that: E =

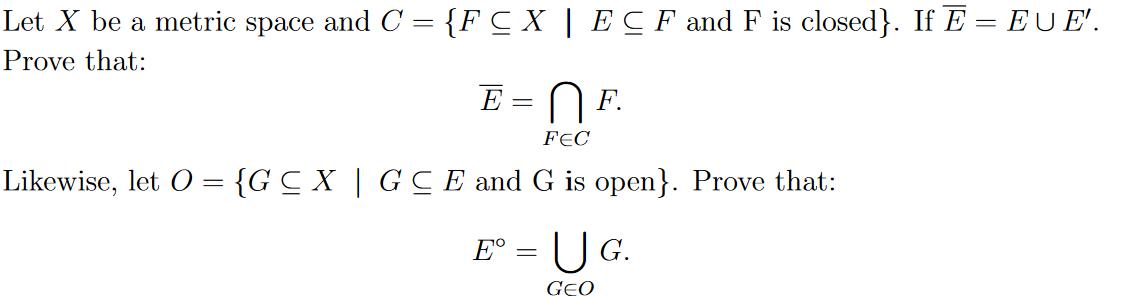
Let X be a metric space and C = {FCX | ECF and F is closed}. If = EU E'. Prove that: E = F. FEC Likewise,

Let X be a metric space and C = {FCX | ECF and F is closed}. If E = EUE'. Prove that: E = F. FEC Likewise, let O = {GCX | G C E and G is open}. Prove that: E = UG. GEO

Step by Step Solution

★★★★★

3.52 Rating (169 Votes )

There are 3 Steps involved in it

Step: 1

To prove the given statements we will use the definitions and properties of closed sets and open sets in a metric space 1 Proving E F Lets start by pr... blur-text-image

Get Instant Access to Expert-Tailored Solutions

See step-by-step solutions with expert insights and AI powered tools for academic success

Step: 2

blur-text-image

Step: 3

blur-text-image

Ace Your Homework with AI

Get the answers you need in no time with our AI-driven, step-by-step assistance

Get Started

Recommended Textbook for

An Introduction to Analysis

An Introduction to Analysis

Authors: William R. Wade

4th edition

132296381, 978-0132296380

More Books

Students also viewed these Accounting questions

Question

★★★★★
Let X be a metric space. a) Prove that if E X is compact, then E is sequentially compact (see Exercise 10.1.10). b) Prove that if X is separable and satisfies the Bolzano-Weierstrass Property, then...

Answered: 1 week ago

Question

★★★★★
Let X be a metric space. Prove that X is connected if and only if every nonempty proper subset of X has a nonempty boundary.

Answered: 1 week ago

Question

★★★★★
Prove Theorem 6.2.7. In Theorem 6.2.7 Chernoff Bounds. Let X be a random variable with moment generating function Ï. Then, for every real t, Pr(X > t) Answered: 1 week ago

Answered: 1 week ago

Question

★★★★★
In Exercises 35 through 42, the slope f'(x) at each point (x, y) on a curve y = f(x) is given along with a particular point (a, b) on the curve. Use this information to find f(x). f'(x) = 3x 2 + 6x ...

Answered: 1 week ago

Question

★★★★★
1. If Siler Chapman had so much trouble running three restaurants as an independent owner, why do you think he was able to manage so many more as a franchisee?\ 2. From a franchisors perspective, why...

Answered: 1 week ago

Question

★★★★★
Gourmet Fast Foods produces and sells many products in each of its 35 different product lines. Occasionally, a product or an entire product line is dropped because it ceases to be profitable. The...

Answered: 1 week ago

Question

★★★★★
In the article Zinc gluconate lozenges for treating the common cold in children: a randomized controlled trial published in the Journal of the American Medical Association (Macknin et al., 1998), the...

Answered: 1 week ago

Question

★★★★★
The Foreman Companys earnings and common stock dividends have been growing at an annual rate of 6 percent over the past 10 years and are expected to continue growing at this rate for the foreseeable...

Answered: 1 week ago

Question

★★★★★
How can you evaluate an app for ethical design?

Answered: 1 week ago

Question

★★★★★
Garnett Jackson, the founder and CEO of Tech Tune-Ups, stared out the window as he finished his customary peanut butter and jelly sandwich, contemplating the dilemma currently facing his firm. Tech...

Answered: 1 week ago

Question

★★★★★
Performance standards are set with respect to which of the following? (Check all that apply.) Multiple select question. cost time used authority quantity measurement quality

Answered: 1 week ago

Question

★★★★★
The optical system of Figure P33.95 consists of a converging lens and a converging mirror. The focal points of the lens are marked by open dots and the center of curvature of the mirror by a solid...

Answered: 1 week ago

Question

★★★★★
In testing the cash discounts on vendors invoices, the auditor did not expect to find any errors in the sample and therefore used attributes sampling techniques. Based upon the statistical...

Answered: 1 week ago

Question

★★★★★
A gift shop sells 400 boxes of scented candles a year. The ordering cost is \($60\) for scented candles, and holding cost is \($24\) per box per year. What is the minimum annual amount of these...

Answered: 1 week ago

Question

★★★★★
A car rental agency uses 96 boxes of staples a year. The boxes cost \($4\) each. It costs \($10\) to order staples, and it costs \($0.80\) to hold a box of staples in inventory for 1 year. How many...

Answered: 1 week ago

Question

★★★★★
How do you add two harmonic motions having different frequencies?

Answered: 1 week ago

Question

★★★★★
Classic Company designs, markets, and distributes a variety of apparel, home decor, accessory, and fragrance products. The companys products include such brands as Polo by Classic, Classic Purple...

Answered: 1 week ago

Question

★★★★★
A bar of length = 1 has one fixed and one free end and stiffness function c(x) = 1 - x. Find the displacement when subjected to a unit force. Pay careful attention to the boundary condition at the...

Answered: 1 week ago

Question

★★★★★
Suppose that f : [0, 1] [a, b] is integrable on [0, 1]. Assume that ef(x) and |f(x)|p are integrable for all 0 a) Prove that for all 0 b) If 0 c) State and prove analogues of these results for...

Answered: 1 week ago

Question

★★★★★
Use Exercise 10.7.6 to prove that if f is continuous and periodic on [0. 2Ï] and for k = 0,1,..., then f(x) = 0 for all x [a, b]. 2T f (x) cos(kx) dx = f(x) sin(kx) dx = 0

Answered: 1 week ago

Question

★★★★★
Let f : [a, b] R, a = x0 f(x) dx = SIxk f(x) dx

Answered: 1 week ago

Question

★★★★★
The accompanying data resulted from an experiment to investigate whether yield from a certain chemical process depended either on the formulation of a particular input or on mixer speed. A...

Answered: 1 week ago

Question

★★★★★
Show how a t CI for can be obtained. Then compute a 95% interval for using the data from Exercise 19. [Hint: With , the result of Exercise 24 (a) indicates how to obtain . Then compute and and obtain...

Answered: 1 week ago

Question

★★★★★
To see whether thrust force in drilling is affected by drilling speed (A), feed rate (B), or material used (C), an experiment using four speeds, three rates, and two materials was performed, with two...

Answered: 1 week ago

Previous Question Next Question