Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Let X be a set. Let Teof be the collection of subsets U of X such that U is the empty set or X
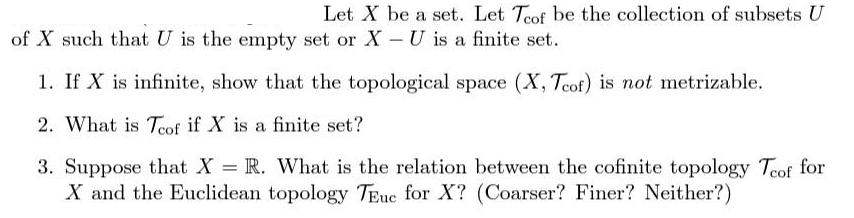
Let X be a set. Let Teof be the collection of subsets U of X such that U is the empty set or X - U is a finite set. 1. If X is infinite, show that the topological space (X, Teof) is not metrizable. 2. What is Teof if X is a finite set? 3. Suppose that X = R. What is the relation between the cofinite topology Teof for X and the Euclidean topology TEuc for X? (Coarser? Finer? Neither?)
Step by Step Solution
★★★★★
3.46 Rating (149 Votes )
There are 3 Steps involved in it
Step: 1
mo no metric space whose topology coincides with Teot chich ix not a property of metsizab...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Discrete Mathematics and Its Applications
Authors: Kenneth H. Rosen
7th edition
0073383090, 978-0073383095
