Question
Let X have a uniform distribution on the interval (0, 1). Given X = x, let Y have a uniform distribution on (0, x). (a)TheconditionalpdfofY,giventhatX=x,isfY|X(y|x)=1
Let X have a uniform distribution on the interval (0, 1). Given X = x, let Y have
a uniform distribution on (0, x). (a)TheconditionalpdfofY,giventhatX=x,isfY|X(y|x)=1 for0 x since Y|X ? U(0,X). Show that the mean of this (conditional) distribution is E(Y |X) = X , and hence, show that EX{E(Y |X)} = 1. [Hint: what is the mean of X ?] 2
5. Let X have a uniform distribution on the interval (0,1). Given X = 3:, let Y have a uniform distribution on (0, :6). (a) The conditional pdf of Y, given that X = :13, is fy|X(y|x) = i for 0 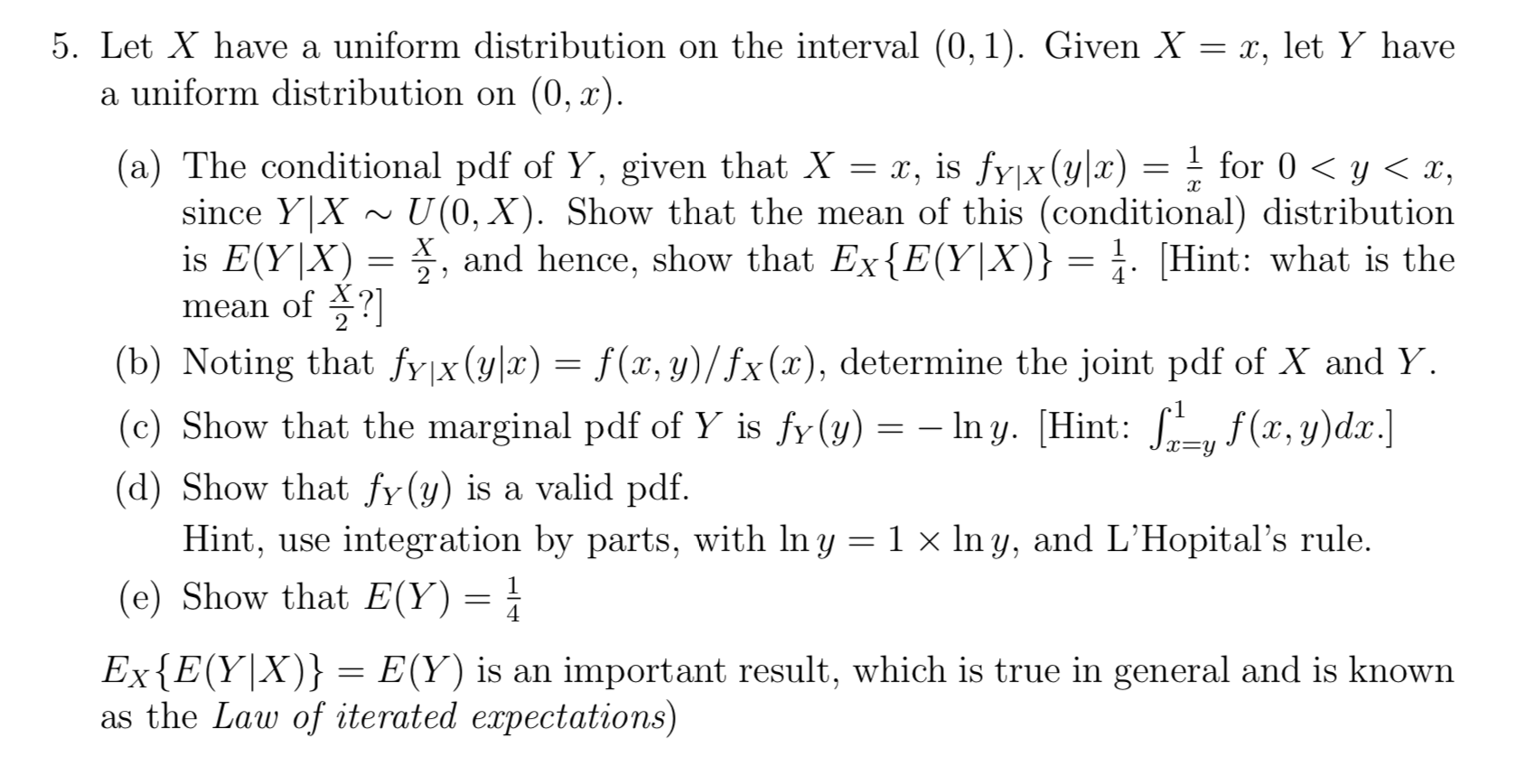
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


